Evidence of Design in Mathematics

Evidence of Design in Mathematics

https://reasonandscience.catsboard.com/t1360-evidence-of-design-in-mathematics

Johannes Kepler, Defundamentis Astrologiae Certioribus, Thesis XX (1601)
"The chief aim of all investigations of the external world should be to discover the rational order and harmony which has been imposed on it by God and which He revealed to us in the language of mathematics."
https://web.archive.org/web/20110805203154/http://www.leaderu.com/real/ri9403/evidence.html

There does exist a world (of universals or the form of the Good, which you can identify with God), which transcends the physical empirical world, and this world of intelligible forms is responsible for the “enforcement” of mathematical order in the physical world. Thus, intelligibility is responsible for the physical world.

The other theory of mathematics, the one which I happen to favour because it doesn’t require such icky occultic philosophical entities, is the idea that mathematics is a human creation. Just like how we invented chess, likewise did we humans invent mathematics. But even though chess and mathematics is our invention, but once invented it has a reality independent of our subjectivity. Thus, for example, it is an objectively true fact that one is able to checkmate in a number of moves, or one is forced to make a certain move to get out of a check, etc. The analogy which Karl Popper used is that of a spider spinning a web. The spider made the web, but once the web was made, it has an objective reality of its own, it is of a certain biochemistry, of a certain pattern, structure in order to retain its integrity, etc.

Thus, mathematics is a product of our minds, in exactly the same way that chess, fictional stories, myths, musical compositions, etc, are products of our minds. Thus, upon this conception, the miracle is that the universe happens to conform to our mind generated realities, that the universe is governed, structured, ordered by a mind generated reality. Therefore we can infer the universe is in fact ordered by a like mind upon the basis of the mind-resonating, that is, resonance and conformity to mind generated realities of mathematics, which the universe possesses.

The naturalistic explanation only explains how we came to create those mental constructs which are able to represent the universe, but it doesn’t explain why does nature itself possess those cognitive resonating and conformity to mind-like orders in the first place.

Perhaps the most convincing evidence that the world was expressly designed to conform to simple laws that man would readily discover is furnished by the universal law of gravitation: F= Gm[1]m[2]/r^2. Notice the exponent 2. Why is it not 1.9999999 . . ., or 4.3785264 . . ., or something else hard to use in computations? Yet research has been able to specify the exponent as far as the first six digits, giving 2.00000. Thus, so far as we can tell, the exponent is exactly 2. Coulomb's law of electric force is similar: F = kq[1]q[2]/r^2. In this case, research has established that the exponent is no different from exactly 2 as far as the first 17 digits. Would we find such laws in an accidental universe?

Matter-antimatter pair production ratio 1 in 10 billion of “leftover particles” happens to be the exact amount of mass necessary for the formation of stars, galaxies, and planets. The number of electrons (in the universe) is equivalent to the number of protons to an accuracy of one part in 10 to the 37th power. If it were not so, galaxies, stars, and planets would never form.

Upon the finetuning by a happy accident of the cosmological constant,  the probability that our universe contains galaxies is akin to exactly 10^123. That is 1 possibility in 1,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000, 000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000 .

The Earth is 366.3% the diameter of the Moon and the Moon is 27.3% the diameter of Earth. 366.3% x 27.3% = .999999. The combined diameter of all the planets in our solar system is 10 times greater than the Earth’s circumference. This has astonishingly high accuracy at 99.99%. The distance between the moon and the sun is 400 times greater than the distance from the earth and the moon. The Sun happens to be 400 times the Moon’s diameter, and 400 times as far away. This means the Sun and Moon appear to be the same size when viewed from Earth. The circumference of the earth at the equator is 24,901.55 miles. The earth’s rotation speed is 1037.5646 mph. If you divide 24,901.55 by 1037.5646 you get 24. Which is the number of hours in a complete day. Just enough rotation speed compared to it’s size to equal a perfect exposure to both sun light and darkness so that life could exist here.

Albert Einstein in "Geometry and Experience" (1921):
“How can it be that mathematics, being after all a product of human thought which is independent of experience, is so admirably appropriate to the objects of reality?"

Only a tiny fraction of our knowledge and interaction with the world is based on scientific knowledge.
Are our minds a goal or only byproduct?
Max Tegmark: "Nature is clearly giving us hints that the universe is mathematical," many mathematicians even feel that they don't invent mathematical structures, "they just discover them -- that these mathematical structures exist independently of humans."  "If we really believe that nature is fundamentally mathematical, then we should look for mathematical patterns and regularities when we come across phenomena that we don't understand. This problem-solving approach has been at the heart of physics' success for the past 500 years."

The universe, which was created very long before human, is mathematical, geometrical and incredibly harmonized in such a way that the human brain could not ignore this highly organized system.

http://www.themoorings.org/apologetics/theisticarg/teleoarg/teleo2.html
http://rationalityofaith.wordpress.com/2013/04/09/two-types-of-design-argument-on-mathematical-order-and-existential-meanings/

Scientist quotes about God, evolution, intelligent design
https://br.pinterest.com/pin/379639443586585733/

Evidence of Design in Mathematics

https://reasonandscience.catsboard.com/t1360-evidence-of-design-in-mathematics#6485

IN 1913, THE DANISH physicist Niels Bohr formulated a new theory of the actions of electrons as they orbited the nucleus of the atom, a critical step towards the development of quantum mechanics in the 1920s, leading to his winning the Nobel prize in physics in 1922. He continued to be among the most influential figures among the physicists of the world for decades to come, and is today commonly ranked among the greatest physicists of history. Like a number of other leading physicists of the twentieth century, Bohr also had a deep interest in the implications of the discoveries of twentieth-century physics for the overall understanding of the human situation in the world. He recognized that physics in its radically new portrayal of the workings of nature was also raising deep philosophical and religious questions. In his 1938 essay, “Natural Philosophy and Human Cultures,” an address delivered to the International Congress of Anthropological and Ethnological Sciences, he wrote that: 

 “it is impossible to distinguish sharply between natural philosophy” as developed by physics “and human culture. The physical sciences are, in fact, an integral part of our civilization, not only because our ever-increasing mastery of nature has so completely changed the material conditions of life, but also because the study of these sciences has contributed so much to clarify the background of our own existence” as human beings. It was thus of great significance that twentieth-century physics had required a “revision of the presuppositions underlying the unambiguous use of even our most elementary concepts such as space and time.” Indeed, “in the study of atomic phenomena we have [now] repeatedly been taught that questions which were believed to have received long ago their final answers had most unexpected surprises in store for us.” Of particular importance, it was no longer possible “to distinguish sharply between the behavior of [physical] objects and the means of [their] observation,” as historically was the view of physicists (and most other people). Since ultimately the means of observation are inseparable from the workings of the human sensory organs and human consciousness, this newly linked what had previously been two distinct fields of investigation, the physics of the natural world and the psychology of human consciousness. Bohr would thus write that the scientific questions in the two areas resemble one another in that there is a “close analogy between the situation as regards the analysis of atomic phenomena . . . and characteristic features of the problem of observation in human psychology.” It is impossible for us to describe, as Bohr comments, in an entirely objective manner our own “psychical experiences . . . such as ‘thoughts’ and ‘feelings’” because we combine inseparably in our human consciousness the roles of observer and observed. In atomic physics, it was surprisingly similar in that there is a “complementary relationship . . . regarding the behavior of atoms obtained under different experimental arrangements” in which the observer becomes an integral part of what is actually observed.

The world of mathematics is “supernatural” in that it already exists outside nature, prior to and outside of any material realities in the natural world. Moreover, this supernatural mathematical order somehow—and of this we still have no clue as to how it works—serves to govern the full detailed workings of the entire “physical world”. A governing mathematical intelligence is thus a supernatural, superhuman entity which we call God. If we then choose to define a god as such a supernatural entity having a fully developed mathematical intelligence, we can then say that at least one god very probably exists, a god of mathematical truth that rules the physical world.

At the age of twenty-one, a Russian mathematical prodigy, Edward Frenkel, still short of having earned his undergraduate degree in Moscow, was invited in 1989 to be a visiting professor of mathematics at Harvard University. Very much impressed with his abilities, Harvard invited Frenkel to remain at Harvard, and he obtained a PhD in mathematics there in one year, and then joined the Harvard faculty. He moved to the University of California at Berkeley in 1997 where he today ranks among the leading mathematicians in the world.

Following others, Frenkel clearly and explicitly recognizes that this view of the universe can be traced back to Plato—that the Platonic mathematical world exists altogether “independent of physical reality.” One of the most important mathematical findings of the nineteenth century, for example, “Galois groups were discovered by the French prodigy [in the 1830s], not invented by him. Until he did so, this concept lived somewhere in the enchanted gardens of the ideal world of mathematics, waiting to be found” by some human being. If it had not been Galois, it would have been someone else later who would have made the same discovery because a mathematical truth is an objective fact, even as it has no physical reality. As Frenkel affirms, “mathematical entities are independent of our rational faculties” as human beings and thus “mathematical truths are inevitable.” The objective truth that two plus two equals four precedes any human existence and will be true for eternity, whatever happens to human beings. Frenkel is confident that in thinking this way he has a great deal of company among contemporary mathematicians and physicists. Indeed, he finds that “most math practitioners believe that mathematical formulas and ideas inhabit a separate world.” He notes that “Kurt Godel, whose work . . . revolutionized mathematical logic, was an unabashed proponent of this view. He wrote that mathematical concepts ‘form an objective reality of their own, which we cannot create or change, but only perceive and describe.’” In the nineteenth century, as Frenkel observes, the physicist “Heinrich Hertz, who proved the existence of magnetic waves, . . . expressed his awe this way: One cannot escape the feeling that these mathematical formulas have an independent existence and intelligence of their own, that they are wiser than we are, wiser than their discoverers.” They are, as such language suggests, similar to if not identical to a god.

By way of illustration, Frenkel comments that “the discovery of quarks” in the 1960s “is a good example of the paramount role played by mathematics in science.” Quarks “were predicted not on the basis of physical data, but on the basis of mathematical symmetry patterns.” Physicists then made an educated guess that certain physical phenomena would later be found to correspond to these mathematical patterns. It turned out in this case, as in many other great scientific discoveries, to be true. As Frenkel explains, “this was a purely theoretical prediction, made within the framework of a sophisticated mathematical theory of representation of the group SU.” In developing the physics of quarks, “a seemingly esoteric mathematical theory empowered us to get to the heart of the building blocks of nature.” As Frenkel puts it, “How can we not be enthralled by the magic harmony of these tiny blobs of matter, not marvel at the capacity of mathematics to reveal the inner workings of the universe?” Truth be told, there is no other way to see it other than as the manifestation of something supernatural.

As Wigner pointed out and Frenkel now again affirms, there are thus two miracles to be confronted, first the magical existence of a world of mathematical truths independent of human existence and physical reality, and second the magical correspondence of at least some of these mathematical truths to the “physical” workings of the natural world (as we perceive them in human consciousness). No one has any scientific explanation for any of this. As Frenkel comments about the total mystery, although physicists and other “scientists have been exploiting this ‘effectiveness’ [of mathematics] for centuries, its roots are still poorly understood”—indeed, are not really understood at all, as Frenkel would likely concede, if pressed on the matter.

There is no explicit mention of a god or explicit discussion of theology in Love & Math. But the book is nevertheless an important work of theology for our time. Contemporary naturalists take for granted that matter must be fundamental, since physical science—for them the one all-powerful method of understanding the world—is all about explaining the workings of the material world. Frenkel is not the first to do so but he offers a strong up-to-date statement from a world-class contemporary mathematician that mathematics is more fundamental than matter. This is all based on a rational analysis as he develops it, employing the skills in reasoning that are found at the highest levels among mathematicians. Indeed, while Frenkel may not realize it himself, he is laying out a rational argument for the very probable existence of a god.

Many mathematicians and physicists believe that the mathematical world has an objective reality outside any physical domain of time and space.

Max Tegmark: 
“we don’t invent mathematical structures—we discover them, and invent only the notation for describing them.”

They simply exist in a realm of abstract ideas and have always existed there. Mathematicians (or physicists doing mathematics) can visit this realm through their own heroic rational efforts within human consciousness. The role of physicists is then to search for exact correlations between such mathematical ideas and the behaviour of the physical world—again as it is necessarily perceived in their consciousness.

Seemingly in a miraculous fashion, even though all this necessarily takes place within the consciousness of each individual physicist, in practice the community of all the physicists of the world can often reach a consensus, establishing a worldwide common agreement on these laws—something altogether impossible outside the physical sciences. It is yet another piece of evidence that a supernatural god must lie somewhere behind all this—what else could be the explanation?

At the deepest level of understanding of reality (the goal of Tegmark’s inquiry), therefore, with respect to the physical world there is seemingly nothing but the mathematics itself, plus the human “baggage” of words—the “poetry” of physics—that are added by physicists to aid heuristically in their own thinking and in communicating their results to others. But if we want to discover the deepest reality that transcends any human linguistic additions, it will be necessary to limit our claims to an understanding of the mathematics itself. Hence, as Tegmark concludes, if we are to seek a physical reality that is independent of any human influence, it follows that such an “external reality is a mathematical structure.” In other words, “something that has a complete baggage-free description” outside human language “is precisely a mathematical structure” in and of itself alone.

Since the mathematical world and its rational truths have always existed, even before the arrival of human beings on earth, the mathematics itself becomes the ultimate bedrock of the reality of the universe. Tegmark, like Wigner, Penrose, and other physicists today, admittedly has no way of explaining why our perceptions of a “physical” world in our human consciousness (as often aided by measuring devices) have turned out to correlate so exactly with one or another eternal mathematical truth that human beings have rationally discovered and explored. It may be all part of the giant mystery of the universe that seemingly only a god could reveal.

The nonphysical workings of human consciousness remain well outside our physical scientific understanding at present. Human consciousness as yet another “internal reality” (besides the physical and mathematical realities) that consists of “the way you subjectively perceive the external reality from the internal vantage point of your mind.” This third internal reality of human consciousness— Tegmark acknowledges some similarities to the three-world views of fellow physicist Roger Penrose —“exists only internally to you; your mind feels as though it’s looking at the outside world, while its only looking at a reality model inside your head.”




1. God? Very Probably: Five Rational Ways to Think about the Question of a God

Blueprint for a Habitable Universe - Mathematics and the Deep Structure of the Universe 1

Johannes Kepler, Defundamentis Astrologiae Certioribus, Thesis XX (1601)
"The chief aim of all investigations of the external world should be to discover the rational order and harmony which has been imposed on it by God and which He revealed to us in the language of mathematics."

Table 1. The Fundamental Laws of Nature.

Yet even the splendid orderliness of the cosmos, expressible in the mathematical forms seen in Table 1, is only a small first step in creating a universe with a suitable place for habitation by complex, conscious life. The particulars of the mathematical forms themselves are also critical. Consider the problem of stability at the atomic and cosmic levels. Both Hamilton's equations for non-relativistic, Newtonian mechanics and Einstein's theory of general relativity (see Table 1) are unstable for a sun with planets unless the gravitational potential energy is proportional to r^-1, a requirement that is only met for a universe with three spatial dimensions. For Schrödinger's equations for quantum mechanics to give stable, bound energy levels for atomic hydrogen (and by implication, for all atoms), the universe must have no more than three spatial dimensions. Maxwell's equations for electromagnetic energy transmission also require that the universe be no more than three-dimensional.


Richard Courant illustrates this felicitous meeting of natural laws with the example of sound and light: "[O]ur actual physical world, in which acoustic or electromagnetic signals are the basis of communication, seems to be singled out among the mathematically conceivable models by intrinsic simplicity and harmony."{8}


To summarize, for life to exist, we need an orderly (and by implication, intelligible) universe. Order at many different levels is required. For instance, to have planets that circle their stars, we need Newtonian mechanics operating in a three-dimensional universe. For there to be multiple stable elements of the periodic table to provide a sufficient variety of atomic "building blocks" for life, we need atomic structure to be constrained by the laws of quantum mechanics. We further need the orderliness in chemical reactions that is the consequence of Boltzmann's equation for the second law of thermodynamics. And for an energy source like the sun to transfer its life-giving energy to a habitat like Earth, we require the laws of electromagnetic radiation that Maxwell described.


Our universe is indeed orderly, and in precisely the way necessary for it to serve as a suitable habitat for life. The wonderful internal ordering of the cosmos is matched only by its extraordinary economy. Each one of the fundamental laws of nature is essential to life itself. A universe lacking any of the laws shown in Table 1 would almost certainly be a universe without life. Many modern scientists, like the mathematicians centuries before them, have been awestruck by the evidence for intelligent design implicit in nature's mathematical harmony and the internal consistency of the laws of nature. Australian astrophysicist Paul Davies declares:

All the evidence so far indicates that many complex structures depend most delicately on the existing form of these laws. It is tempting to believe, therefore, that a complex universe will emerge only if the laws of physics are very close to what they are....The laws, which enable the universe to come into being spontaneously, seem themselves to be the product of exceedingly ingenious design. If physics is the product of design, the universe must have a purpose, and the evidence of modern physics suggests strongly to me that the purpose includes us.{9}

British astronomer Sir Fred Hoyle likewise comments,

I do not believe that any scientist who examines the evidence would fail to draw the inference that the laws of nuclear physics have been deliberately designed with regard to the consequences they produce inside stars. If this is so, then my apparently random quirks have become part of a deep-laid scheme. If not then we are back again at a monstrous sequence of accidents.{10}

Nobel laureates Eugene Wigner and Albert Einstein have respectfully evoked "mystery" or "eternal mystery" in their meditations upon the brilliant mathematical encoding of nature's deep structures. But as Kepler, Newton, Galileo, Copernicus, Davies, and Hoyle and many others have noted, the mysterious coherency of the mathematical forms underlying the cosmos is solved if we recognize these forms to be the creative intentionality of an intelligent creator who has purposefully designed our cosmos as an ideal habitat for us.

Blueprint for a Habitable Universe: Universal Constants - Cosmic Coincidences?

Next, let us turn to the deepest level of cosmic harmony and coherence - that of the elemental forces and universal constants which govern all of nature. Much of the essential design of our universe is embodied in the scaling of the various forces, such as gravity and electromagnetism, and the sizing of the rest mass of the various elemental particles such as electrons, protons, and neutrons.
There are certain universal constants that are indispensable for our mathematical description of the universe (see Table 2). These include Planck's constant, h; the speed of light, c; the gravity-force constant, G; the rest masses of the proton, electron, and neutron; the unit charge for the electron or proton; the weak force, strong nuclear force, electromagnetic coupling constants; and Boltzmann's constant, k.

Speed of light	c = 3.0 x 108 m/s
Planck's constant	h = 6.63 x 10-34 J-s
Boltzmann's constant	k = 1.38 x 10-23 J / oK
Unit charge	q = 1.6 x 10-19 Coulombs
Rest mass proton	mp = 1.67 x 10-27 kg
Rest mass of neutron	mn = 1.69 x 10-27 kg
Rest mass of electron	me = 9.11 x 10-31 kg
gravity force constant	G = 6.67 x 10-11 N-m2/ kg2

When cosmological models were first developed in the mid-twentieth century, cosmologists naively assumed that the selection of a given set of constants was not critical to the formation of a suitable habitat for life. Through subsequent parametric studies that varied those constants, scientists now know that relatively small changes in any of the constants produce a dramatically different universe and one that is not hospitable to life of any imaginable type.
The "just so" nature of the universe has fascinated both scientists and laypersons, giving rise to a flood of titles such as The Anthropic Cosmological Principle,{11} Universes,{12} The Accidental Universe,{13} Superforce,{14} The Cosmic Blueprint,{15} Cosmic Coincidences,{16} The Anthropic Principle,{17} Universal Constants in Physics,{18} The Creation Hypothesis,{19} and Mere Creation: Science, Faith and Intelligent Design.{20} Let us examine several examples from a longer list of approximately one hundred requirements that constrain the selection of the universal constants to a remarkable degree.
Twentieth-century physicists have identified four fundamental forces in nature. These may each be expressed as dimensionless numbers to allow a comparison of their relative strengths. These values vary by a factor of 10⁴¹ (10 with forty additional zeros after it), or by 41 orders of magnitude. Yet modest changes in the relative strengths of any of these forces and their associated constants would produce dramatic changes in the universe, rendering it unsuitable for life of any imaginable type. Several examples to illustrate this fine-tuning of our universe are presented next.
Balancing Gravity and Electromagnetism Forces - Fine Tuning Our Star and Its Radiation
The electromagnetic force is 10³⁸ times stronger than the gravity force. Gravity draws hydrogen into stars, creating a high temperature plasma. The protons in the plasma must overcome their electromagnetic repulsion to fuse. Thus the relative strength of the gravity force to the electromagnetic force determines the rate at which stars "burn" by fusion. If this ratio of strengths were altered to10³² instead of 10³⁸ (i.e., if gravity were much stronger), stars would be a billion times less massive and would burn a million times faster.{21}
Electromagnetic radiation and the light spectrum also depend on the relative strengths of the gravity and electromagnetic forces and their associated constants. Furthermore, the frequency distribution of electromagnetic radiation produced by the sun must be precisely tuned to the energies of the various chemical bonds on Earth. Excessively energetic photons of radiation (i.e., the ultraviolet radiation emitted from a blue giant star) destroy chemical bonds and destabilize organic molecules. Insufficiently energetic photons (e.g., infrared and longer wavelength radiation from a red dwarf star) would result in chemical reactions that are either too sluggish or would not occur at all. All life on Earth depends upon fine-tuned solar radiation, which requires, in turn, a very precise balancing of the electromagnetic and gravitational forces.
As previously noted, the chemical bonding energy relies upon quantum mechanical calculations that include the electromagnetic force, the mass of the electron, the speed of light (c), and Planck's constant (h). Matching the radiation from the sun to the chemical bonding energy requires that the magnitude of six constants be selected to satisfy the following inequality, with the caveat that the two sides of the inequality are of the same order of magnitude, guaranteeing that the photons are sufficiently energetic, but not too energetic.{22}
Substituting the values in Table 2 for h, c, G, m_e, m_p, and e (with units adjusted as required) allows Equation 3 to be evaluated to give:
In what is either an amazing coincidence or careful design by an intelligent Creator, these constants have the very precise values relative to each other that are necessary to give a universe in which radiation from the sun is tuned to the necessary chemical reactions that are essential for life. This result is illustrated in Figure 3, where the intensity of radiation from the sun and the biological utility of radiation are shown as a function of the wavelength of radiation. The greatest intensity of radiation from the sun occurs at the place of greatest biological utility.

(Figure 3.1.)

(Figure 3.2.)

(Figure 3.3.)

(Figure 3.4.)
Figure 3. The visible portion of the electromagnetic spectrum (~1 micron) is the most intense radiation from the sun (Figure 3.1); has the greatest biological utility (Figure 3.2); and passes through atmosphere of Earth (Figure 3.3) and water (Figure 3.4) with almost no absorption. It is uniquely this same wavelength of radiation that is idea to foster the chemistry of life. This is either a truly amazing series of coincidences or else the result of careful design.
Happily, our star (the sun) emits radiation (light) that is finely tuned to drive the chemical reactions necessary for life. But there is still a critical potential problem: getting that radiation from the sun to the place where the chemical reactions occur. Passing through the near vacuum of space is no problem. However, absorption of light by either Earth's atmosphere or by water where the necessary chemical reactions occur could render life on Earth impossible. It is remarkable that both the Earth's atmosphere and water have "optical windows" that allow visible light (just the radiation necessary for life) to pass through with very little absorption, whereas shorter wavelength (destructive ultraviolet radiation) and longer wavelength (infrared) radiation are both highly absorbed, as seen in Figure 3.{23} This allows solar energy in the form of light to reach the reacting chemicals in the universal solvent, which is water. The Encyclopedia Britannica{24} observes in this regard:

Considering the importance of visible sunlight for all aspects of terrestrial life, one cannot help being awed by the dramatically narrow window in the atmospheric absorption...and in the absorption spectrum of water.

It is remarkable that the optical properties of water and our atmosphere, the chemical bonding energies of the chemicals of life, and the radiation from the sun are all precisely harmonized to allow living systems to utilize the energy from the sun, without which life could not exist. It is quite analogous to your car, which can only run using gasoline as a fuel. Happily, but not accidentally, the service station has an ample supply of exactly the right fuel for your automobile. But someone had to drill for and produce the oil, someone had to refine it into liquid fuel (gasoline) that has been carefully optimized for your internal combustion engine, and others had to truck it to your service station. The production and transportation of the right energy from the sun for the metabolic motors of plants and animals is much more remarkable, and hardly accidental.
Finally, without this unique window of light transmission through water, which is constructed upon an intricate framework of universal constants, vision would be impossible and sight-communication would cease, since living tissue and eyes are composed mainly of water.
Nuclear Strong Force and Electromagnetic Force - Finely Balanced for a Universe Rich in Carbon and Oxygen (and therefore water)
The nuclear strong force is the strongest force within nature, occurring at the subatomic level to bind protons and neutrons within atomic nuclei.{25} Were we to increase the ratio of the strong force to the electromagnetic force by only 3.4 percent, the result would be a universe with no hydrogen, no long-lived stars that burn hydrogen, and no water (a molecule composed of two hydrogen atoms and one oxygen atom)--our "universal solvent" for life. Likewise, a decrease of only 9 percent in the strong force relative to the electromagnetic force would decimate the periodic table of elements. Such a change would prevent deuterons from forming from the combination of protons and neutrons. Deuterons, in turn, combine to form helium, then helium fuses to produce beryllium, and so forth.{26}
Within the nucleus, an even more precise balancing of the strong force and the electromagnetic force allows for a universe with an abundance of organic building blocks, including both carbon and oxygen.{27} Carbon serves as the universal connector for organic life and is an optimal reactant with almost every other element, forming bonds that are stable but not too stable, allowing compounds to be formed and disassembled. Oxygen is a component of water, the necessary universal solvent where life chemistry can occur. This is why when people speculate about life on Mars, they first look for signs of organic molecules (ones containing carbon) and signs that Mars once had water.
Quantum physics examines the most minute energy exchanges at the deepest levels of the cosmic order. Only certain energy levels are permitted within nuclei-like steps on a ladder. If the mass-energy for two colliding particles results in a combined mass-energy that is equal to or slightly less than a permissible energy level on the quantum "energy ladder," then the two nuclei will readily stick together or fuse on collision, with the energy difference needed to reach the step being supplied by the kinetic energy of the colliding particles. If this mass-energy level for the combined particles is exactly right, then the collisions are said to have resonance, which is to say that there is a high efficiency within the collision. On the other hand, if the combined mass-energy results in a value that is slightly higher than one of the permissible energy levels on the energy ladder, then the particles will simply bounce off each other rather than fusing, or sticking together.
It is clear that the step sizes between quantum nuclear energy levels depends on the balance between the strong force and the electromagnetic force, and these steps must be tuned to the mass-energy levels of various nuclei for resonance to occur and give an efficient conversion by fusion of lighter element into carbon, oxygen and heavier elements.
In 1953, Sir Fred Hoyle et al. predicted the existence of the unknown resonance energy level for carbon, and it was subsequently confirmed through experimentation.{28} In 1982, Hoyle offered a very insightful summary of the significance he attached to his remarkable predictions.

From 1953 onward, Willy Fowler and I have always been intrigued by the remarkable relation of the 7.65 MeV energy level in the nucleus of 12 C to the 7.12 MeV level in 16 O. If you wanted to produce carbon and oxygen in roughly equal quantities by stellar nucleosynthesis, these are the two levels you would have to fix, and your fixing would have to be just where these levels are actually found to be. Another put-up job? Following the above argument, I am inclined to think so. A common sense interpretation of the facts suggests that a super intellect has "monkeyed" with the physics as well as the chemistry and biology, and there are no blind forces worth speaking about in nature.{29}

The Rest Mass of Subatomic Particles - Key to Universe Rich in Elemental Diversity
Scientists have been surprised to discover the extraordinary tuning of the masses of the elementary particles to each other and to the forces in nature. Stephen Hawking has noted that the difference in the rest mass of the neutron and the rest mass of the proton must be approximately equal to twice the mass of the electron. The mass-energy of the proton is 938.28 MeV and the mass-energy of the neutron is 939.57 MeV. The mass-energy of the electron is 0.51 MeV, or approximately half of the difference in neutron and proton mass-energies, just as Hawking indicated it must be.{30} If the mass-energy of the proton plus the mass-energy of the electron were not slightly smaller than the mass-energy of the neutron, then electrons would combine with protons to form neutrons, with all atomic structure collapsing, leaving an inhospitable world composed only of neutrons.
On the other hand, if this difference were larger, then neutrons would all decay into protons and electrons, leaving a world of pure hydrogen, since neutrons are necessary for protons to combine to build heavier nuclei and the associated elements. As things stand, the neutron is just heavy enough to ensure that the Big Bang would yield one neutron to every seven protons, allowing for an abundant supply of hydrogen for star fuel and enough neutrons to build up the heavier elements in the universe.{31} Again, a meticulous inner design assures a universe with long-term sources of energy and elemental diversity.
The Nuclear Weak Coupling Force - Tuned to Give an Ideal Balance Between Hydrogen (as Fuel for Sun) and Heavier Elements as Building Blocks for Life
The weak force governs certain interactions at the subatomic or nuclear level. If the weak force coupling constant were slightly larger, neutrons would decay more rapidly, reducing the production of deuterons, and thus of helium and elements with heavier nuclei. On the other hand, if the weak force coupling constant were slightly weaker, the Big Bang would have burned almost all of the hydrogen into helium, with the ultimate outcome being a universe with little or no hydrogen and many heavier elements instead. This would leave no long-lived stars and no hydrogen-containing compounds, especially water. In 1991, Breuer noted that the appropriate mix of hydrogen and helium to provide hydrogen-containing compounds, long-term stars, and heavier elements is approximately 75 percent hydrogen and 25 percent helium, which is just what we find in our universe.{32}
This is obviously only an illustrative--but not exhaustive--list of cosmic "coincidences." Clearly, the four forces in nature and the universal constants must be very carefully calibrated or scaled to provide a universe that satisfies the key requirements for life that we enumerated in our initial "needs statement": for example, elemental diversity, an abundance of oxygen and carbon, and a long-term energy source (our sun) that is precisely matched to the bonding strength of organic molecules, with minimal absorption by water or Earth's terrestrial atmosphere.
John Wheeler, formerly Professor of Physics at Princeton, in discussing these observations asks:

Is man an unimportant bit of dust on an unimportant planet in an unimportant galaxy somewhere in the vastness of space? No! The necessity to produce life lies at the center of the universe's whole machinery and design.....Slight variations in physical laws such as gravity or electromagnetism would make life impossible.{33}

Blueprint for a Habitable Universe: The Criticality of Initial or Boundary Conditions

As we already suggested, correct mathematical forms and exactly the right values for them are necessary but not sufficient to guarantee a suitable habitat for complex, conscious life. For all of the mathematical elegance and inner attunement of the cosmos, life still would not have occurred had not certain initial conditions been properly set at certain critical points in the formation of the universe and Earth. Let us briefly consider the initial conditions for the Big Bang, the design of our terrestrial "Garden of Eden," and the staggering informational requirements for the origin and development of the first living system.
The Big Bang
The "Big Bang" follows the physics of any explosion, though on an inconceivably large scale. The critical boundary condition for the Big Bang is its initial velocity. If this velocity is too fast, the matter in the universe expands too quickly and never coalesces into planets, stars, and galaxies. If the initial velocity is too slow, the universe expands only for a short time and then quickly collapses under the influence of gravity. Well-accepted cosmological models{34} tell us that the initial velocity must be specified to a precision of 1/10⁶⁰. This requirement seems to overwhelm chance and has been the impetus for creative alternatives, most recently the new inflationary model of the Big Bang.
Even this newer model requires a high level of fine-tuning for it to have occurred at all and to have yielded irregularities that are neither too small nor too large for the formation of galaxies. Astrophysicists originally estimated that two components of an expansion-driving cosmological constant must cancel each other with an accuracy of better than 1 part in 10⁵⁰. In the January 1999 issue of Scientific American, the required accuracy was sharpened to the phenomenal exactitude of 1 part in 10¹²³.{35} Furthermore, the ratio of the gravitational energy to the kinetic energy must be equal to 1.00000 with a variation of less than 1 part in 100,000. While such estimates are being actively researched at the moment and may change over time, all possible models of the Big Bang will contain boundary conditions of a remarkably specific nature that cannot simply be described away as "fortuitous".
The Uniqueness of our "Garden of Eden"
Astronomers F. D. Drake{36} and Carl Sagan{37} speculated during the 1960s and 1970s that Earth-like places in the universe were abundant, at least one thousand but possibly as many as one hundred million. This optimism in the ubiquity of life downplayed the specialness of planet Earth. By the 1980s, University of Virginia astronomers Trefil and Rood offered a more sober assessment in their book, Are We Alone? The Possibility of Extraterrestrial Civilizations.{38} They concluded that it is improbable that life exists anywhere else in the universe. More recently, Peter Douglas Ward and Donald Brownlee of the University of Washington have taken the idea of the Earth's unique place in our vast universe to a much higher level. In their recent blockbuster book, Rare Earth: Why Complex Life is Uncommon in the Universe,{39} they argue that the more we learn about Earth, the more we realize how improbable is its existence as a uniquely habitable place in our universe. Ward and Brownlee state it well:

If some god-like being could be given the opportunity to plan a sequence of events with the expressed goal of duplicating our 'Garden of Eden', that power would face a formidable task. With the best of intentions but limited by natural laws and materials it is unlikely that Earth could ever be truly replicated. Too many processes in its formation involve sheer luck. Earth-like planets could certainly be made, but each would differ in critical ways. This is well illustrated by the fantastic variety of planets and satellites (moons) that formed in our solar system. They all started with similar building materials, but the final products are vastly different from each other . . . . The physical events that led to the formation and evolution of the physical Earth required an intricate set of nearly irreproducible circumstances.{40}

What are these remarkable coincidences that have precipitated the emerging recognition of the uniqueness of Earth? Let us consider just two representative examples, temperature control and plate tectonics, both of which we have alluded to in our "needs statement" for a habitat for complex life.
Temperature Control on Planet Earth
In a universe where water is the primary medium for the chemistry of life, the temperature must be maintained between 0° C and 100° C (32° F to 212° F) for at least some portion of the year. If the temperature on earth were ever to stay below 0° C for an extended period of time, the conversion of all of Earth's water to ice would be an irreversible step. Because ice has a very high reflectivity for sunlight, if the Earth ever becomes an ice ball, there is no returning to the higher temperatures where water exists and life can flourish. If the temperature on Earth were to exceed 100°C for an extended period of time, all oceans would evaporate, creating a vapor canopy. Again, such a step would be irreversible, since this much water in the atmosphere would efficiently trap all of the radiant heat from the sun in a "super-greenhouse effect," preventing the cooling that would be necessary to allow the steam to re-condense to water.{41} This appears to be what happened on Venus.
Complex, conscious life requires an even more narrow temperature range of approximately 5-50° C.{42} How does our portion of real estate in the universe remain within such a narrow temperature range, given that almost every other place in the universe is either much hotter or much colder than planet Earth, and well outside the allowable range for life? First, we need to be at the right distance from the sun. In our solar system, there is a very narrow range that might permit such a temperature range to be sustained, as seen in Fig. 1. Mercury and Venus are too close to the sun, and Mars is too far away. Earth must be within approximately 10% of its actual orbit to maintain a suitable temperature range.{43}
Yet Earth's correct orbital distance from the sun is not the whole story. Our moon has an average temperature of -18° C, while Earth has an average temperature of 33° C; yet each is approximately the same average distance from the sun. Earth's atmosphere, however, efficiently traps the sun's radiant heat, maintaining the proper planetary temperature range. Humans also require an atmosphere with exactly the right proportion of tri-atomic molecules, or gases like carbon dioxide and water vapor. Small temperature variations from day to night make Earth more readily habitable. By contrast, the moon takes twenty-nine days to effectively rotate one whole period with respect to the sun, giving much larger temperature fluctuations from day to night. Earth's rotational rate is ideal to maintain our temperature within a narrow range.


1. https://web.archive.org/web/20110805203154/http://www.leaderu.com/real/ri9403/evidence.html

Most remarkable of all, the sun's radiation has gradually increased in intensity by 40 percent over time--a fact that should have made it impossible to maintain Earth's temperature in its required range. This increase, however, has been accompanied by a gradual decrease in the Earth's concentration of carbon dioxide. Today although the Earth receives more radiation, the atmosphere traps it less efficiently, thus preserving approximately the same temperatures that the Earth experienced four billion years ago. The change in the concentration of carbon dioxide over four billion years has resulted first from plate tectonics (by which carbon dioxide has been converted to calcium carbonate in shallow waters), and more recently through the development of plant life. Such good fortune on such a grand scale must be considered a miracle in its own right. But there is still more to the story.
Mercury, Venus, and Mars all spin on their axes, but their axis angles vary chaotically from 0 to 90 degrees, giving corresponding chaotic variations in their planetary climates. Earth owes its relative climatic stability to its stable 23-degree axis of rotation. This unique stability is somehow associated with the size of Earth's large moon. Our moon is one-third the size of Earth--rare for any planet. To have such a large moon is particularly rare for planets in the inner regions of the solar system, where a habitable temperature range can be sustained. The most current theories explaining this proposition lead us again to the suspicion that such a remarkable and "fortuitous accident" occurred specifically for our benefit.{44}


Figure 4. In our solar system (drawn to scale), notice that the habitable zone is the region within ~10 percent of the orbital radius for planet earth, a very small part of our large, solar system.{43}
Plate Tectonics - Continent Builder, Temperature Controller, Cosmic Radiation Protecter


How does plate tectonics contribute to our planet's becoming habitable for complex life? First, plate tectonics have produced a landmass on an earth that would otherwise have remained a smooth sphere covered by 4000 feet of water. Second, plate tectonics on Earth formed regions of shallow water just beyond the landmass. In these shallows, carbon dioxide chemically reacts with calcium silicate to form calcium carbonate and silicon oxide (or sand). This process removes sufficient carbon dioxide from the atmosphere to avoid overheating as the sun's radiant energy increases. Third, plate tectonics allows for sufficiently large thermal gradients to develop the convective cells in the Earth's core that generate our magnetic field, which in turn protects us from cosmic radiation.
It is reasonable to assume that without plate tectonics, no planet could be habitable.{45} Of the 62 satellites in our solar systems, only Earth has plate tectonic activity--a fact that reflects the difficulty to meet the conditions required for this transformational process. Plate tectonics requires just the right concentration of heavy, radioactive elements in a planet or moon's core, in order to produce the proper amount of heat through radioactive decay. Furthermore, the core must be molten, with a solid, but viscous crust. The viscosity of the crust must be carefully calibrated to the heat generation in the core. The total volume of surface water present on a planet is also critical (on Earth, it is 0.5 percent by weight).{46} Too much water will yield a planet with only oceans. Too little water or too much plate tectonic activity will produce a planet with almost all land mass and very small oceans. This imbalance would leave the Earth with a water cycle that could not aerate the landmass adequately to sustain life. The oceans also buffer temperature fluctuations, helping to keep the Earth's surface temperature in a viable range. Earth's current proportion of 30 percent landmass to 70 percent oceans is biologically ideal. However, this complex end result arises from a myriad of factors that appear to be independent. Again, an explanatory model based on "accidents of nature" seems insufficient to account for yet another remarkable feature of our planet.

Blueprint for Life: Information and The Origin of Life

We have not yet touched on the greatest "miracle" in our terrestrial narrative of origins. While we have noted the remarkable provision of a suitable universe with a local habitat that is ideal for life, the most remarkable artifact in our universe is life itself. While biological evolution, including macroevolution, continues to have a larger constituency than is justified by the evidence (in my opinion), all major researchers in the field of chemical evolution (i.e., the origin of life) acknowledge the fundamental mystery of life's beginnings from inanimate matter. The enigma of the origin of life comes in the difficulty of imagining a simply biological system that is sufficiently complex to process energy, store information, and replicate, and yet at the same time is sufficiently simple to have just "happened" in a warm pond, as Darwin suggested, or elsewhere.
Complex molecules, such as proteins, RNA, and DNA, provide for essential biological functions. These biopolymers are actually long chains of simpler molecular building blocks such as amino acids (of which there are 20 different types--see Figure 5), sugars and bases. Their biological function is intimately connected to their precise chemical structure. How, then, were they assembled with such perfect functionality before the origin of life itself? If I stand across the street and throw paint at my curb, I am not very likely to paint "204," which is my house number. On the other hand, if I first place a template with the numbers "204" on my curb and then sling paint, I can easily paint "204" on my curb. Living systems contain their own templates. However, such templates did not guide the process before life began (i.e., under prebiotic conditions). How, then, did the templates and other molecular machinery originate?


To illustrate the staggering degree of complexity involved here, let us consider a typical protein that is composed of 100 amino acids. Amino acids are molecules that can have two mirror image structures, usually referred to as "left-handed" and "right-handed" variants, as seen in Figure 6. A functional protein requires the amino acids from which it is built to be (1) all left-handed; (2) all linked together with peptide bonds (Figure 7), and (3) all in just the right sequence to fold up into the three-dimensional structure needed for biological function, as seen in Figure 8. The probability of correctly assembling a functional protein in one try in a prebiotic pond, as seen in Figure 8, is 1/10¹⁹⁰.{48} If we took all of the carbon in the universe, converted it into amino acids, and allowed it to chemically react at the maximum permissible rate of 10¹³ interactions per second for five billion years, the probability of making a single functioning protein increases to only 1/10⁶⁰. For this reason, chance explanations for the origin of life have been rejected. Some non-random process or intelligent designer must be responsible. However, there are no apparent nonrandom processes (such as natural selection is claimed to be in evolution) that would seem to be capable of generating the required complexity and information for the first living system.



Figure 5. Schematic of five amino acids. Twenty different amino acids are utilized in protein molecules.



Figure 6. Left- and right-handed versions of amino acids that occur with equal frequency in nature. Only left-handed amino acids are incorporated in protein molecules.



Figure 7. Schematic representation of the formation of peptide bonds with water formed as a byproduct



Figure 8. Schematic representation of the three-dimensional topography of a chain of amino acids. Note shape is critical to biological function.
Making a viable protein from scratch is analogous to writing a sentence in a language with 20 letters in its alphabet (e.g., distinct amino acids), using a random sequencing of the letters as well as random orientations (that is upside down or sideways). Creating a coherent sentence or short paragraph from such a random sequencing of letters strains the imagination. Creating a functioning living system becomes as arduous as writing a long paragraph with such an inefficient approach. These information-generating requirements present the single, greatest obstacle to a purely naturalistic explanation for the origin of life. Researchers in this field are quick to acknowledge this huge problem. For example, Miller and Levine, in their popular textbook, describes the problem as follows:

The largest stumbling block in bridging the gap between nonliving and living still remains. All living cells are controlled by information stored in DNA, which is transcribed in RNA and them made into protein. This is a very complicated system, and each of these three molecules requires the other two--either to put it together or to help it work. DNA, for example, carries information but cannot put that information to use, or even copy itself without the help of RNA and protein.{47}

One of the giants in origin of life research, Leslie Orgel, in a 1998 review entitled The Origin of Life - a review of facts and speculations{48} summarized the current state of affairs with:

There are several tenable theories about the origin of organic material on the primitive earth, but in no case is the supporting evidence compelling. Similarly, several alternative scenarios might account for the self-organization of a self-replicating entity from pre-biotic organic material, but all of those that are well formulated are based on hypothetical chemical syntheses that are problematic.

Nicholas Wade writing in the New York Times (6/13/2000){49} about the origin of life notes:

The chemistry of the first life is a nightmare to explain. No one has yet developed a plausible explanation to show how the earliest chemicals of life - thought to be RNA, or ribonucleic acid, a close relative of DNA, might have constructed themselves from the inorganic chemicals likely to have been around on the early earth. The spontaneous assembly of a small RNA molecule on the primitive earth "would have been a near miracle" two experts in the subject helpfully declared last year.

Interested readers are directed to my more detailed treatment of this topic in a book I co-authored entitled The Mystery of Life's Origin: Reassessing Current Theories.{50}

Do Discoveries of the Last Fifty Years Support Naturalism or Intelligent Design?

My initial example of design was very simple. It involved one physical law, one universal constant, and two initial conditions. These could easily be prescribed so that my water balloon would arrive on the plaza below the Leaning Tower of Pisa just in time to hit my strolling friend. This was a relatively easy design problem.
A universe that contains a special place of habitation for complex, conscious life is so truly remarkable that it is, realistically speaking, impossible to believe it is the result of a series of cosmic accidents. To choose to believe that there is a naturalistic explanation for (a) the mathematical forms encoded in the laws of nature, (b) the precise specification of the nineteen universal constants and (c) the remarkable initial conditions required for star formation and the simplest living systems is to believe in a miracle by another name. Physicist Freeman J. Dyson of Princeton's Institute for Advanced Study seems to implicitly affirm theism when he say,

"As we look out into the universe and identify the many accidents of physics and astronomy that have worked to our benefit, it almost seems as if the universe must in some sense have known that we were coming."{51}

Physicist and Nobel laureate Arno Penzias, contemplating our enigmatic universe, observes:

Astronomy leads us to a unique event, a universe that was created out of nothing and delicately balanced to provide exactly the conditions required to support life. In the absence of an absurdly improbable accident, the observations of modern science seem to suggest an underlying, one might say, supernatural plan.{52}

Astronomer Sir Fred Hoyle argued in The Nature of the Universe{53} in 1950 for the role of sheer coincidence to explain the many unique but necessary properties of the universe and of planet Earth. But the discoveries of the next thirty years dramatically changed his mind, as described in his book The Intelligent Universe in 1983; to quote,

"Such properties seem to run through the fabric of the natural world like a thread of happy coincidences. But there are so many odd coincidences essential to life that some explanation seems required to account for them."{54}

It is easy to understand why many scientists like Sir Fred Hoyle changed their minds in the past thirty years. They now agree that the universe, as we know it, cannot reasonably be explained as a cosmic accident. Frederic B. Burnham, a well-known historian of science appearing on ABC's Nightline with Ted Koppel, confirmed the current openness to the intelligent design model with his comment,

"The scientific community is prepared to consider the idea that God created the universe a more respectable hypothesis today than at any time in the last 100 years."{55}

Concluding Comments

Returning to the Mt. Rushmore illustration with which we began, we must ask ourselves whether our universe and place in it (planet Earth) are more analogous to Mt. Rushmore or to the rock in Hawaii that captures John F. Kennedy's silhouette in its shadow? It seems to me the answer is perfectly clear, based on the myriad of information presented in this paper and the much larger amount of related information in the literature, that the universe is better represented in its complexity by Mt. Rushmore. However, it is worth noting that Mt. Rushmore is a quite inadequate analogy to our universe and habitat in it.
If a few portions of the Mt. Rushmore monument had been made incorrectly, the impressions of the four presidents would not be completely lost, just less accurate. But, if any one of the five fundamental laws of nature is lacking, if any of the universal constants is outside the permissible range of values, or if any of the many initial conditions is not met, then any potential for life in our universe would be obliterated.
The design requirements for our universe are like a chain of 1000 links. If any link breaks, we do not have a less optimal universe for life -- we have a universe incapable of sustaining life! The evidence I have present is daunting, but still short of "proof". I must conclude that it takes a great deal more faith to believe in an accidental universe than to believe in an intelligent creator, or God who crafted such a marvelous universe and beautiful place of habitation in planet Earth, and then created life (including human beings) to occupy it.


Endnotes
{1} William Paley, Natural Theology (London: Wilks and Taylor, 1802).
{2} Richard Dawkins, Climbing Mount Improbable (New York: Norton, 1996), 3.
{3} Johannes Kepler, Defundamentis Astrologiae Certioribus, Thesis XX (1601).
{4} Galileo Galilei, this comment is widely attributed to Galileo, but without reference.
{5} Morris Kline, Mathematics: The Loss of Certainty (New York: Oxford University Press, 1980), 52.
{6} Eugene Wigner, "The Unreasonable Effectiveness of Mathematics in the Physical Sciences," Communications on Pure and Applied Mathematics, vol. 13 (1960): 1-14.
{7} Albert Einstein, Letters to Solovine (New York: Philosophical Library, 1987), 131.
{8} Richard Courant, Partial Differential Equations, Vol. II of R. Courant and D. Hilbert, Methods of Mathematical Physics (New York: Interscience Publishers, 1962), 765-66.
{9} Paul Davies, Superforce (New York: Simon and Schuster, 1984), 243.
{10} Fred Hoyle, Religion and the Scientists, quoted in John Barrow and Frank Tipler, The Anthropic Cosmological Principle (Oxford: Clarendon Press, 1988), 22.
{11} John Barrow and Frank Tipler, The Anthropic Cosmological Principle (Oxford: Clarendon Press, 1988).
{12} John Leslie, Universes (New York: Routledge, 1989).
{13} Paul Davies, The Accidental Universe (Cambridge: Cambridge University Press, 1982).
{14} Paul Davies, Superforce (Portsmouth, N.H.: Heinemann, 1984).
{15} Paul Davies, The Cosmic Blueprint (Portsmouth, N.H.: Heinemann, 1988).
{16} John Gribbin and Martin Rees, Cosmic Coincidences (New York: Bantam Books, 1989).
{17} Reinhard Breuer, The Anthropic Principle, trans. Harry Newman and Mark Lowery (Boston: Birkhäuser, 1991).
{18} Gilles Cohen-Tannoudji, Universal Constants in Physics, trans. Patricia Thickstun (New York: McGraw-Hill, 1993).
{19} J. P. Moreland, ed., The Creation Hypothesis (Downers Grove, Ill.: InterVarsity Press, 1994).
{20} William A. Dembski, Ed. Mere Creation: Science, Faith & Intelligent Design. (Downers Grove, Ill.: InterVarsity Press, 1998).
{21} John Leslie, Universes (New York: Routledge,1989), 36-39.
{22} John Barrow and Frank Tipler, The Anthropic Cosmological Principle, 336.
{23} Michael J. Denton, Nature's Destiny: How the Laws of Biology Reveal Purpose in the Universe (New York: Simon and Schuster, 1998), 56-57.
{24} Encyclopedia Britannica (1994), 15th ed., Vol. 18, 200.
{25} Barrow and Tipler, Anthropic Cosmological Principle, 322.
{26} I.L. Rozental, On Numerical Values of Fundamental Constants (Moscow: 1980), 9.
{27} John Leslie, Universes, 35-40.
{28} F. Hoyle, D.N.F. Dunbar, W.A. Wensel, and W. Whaling, Phys. Rev. 92 (1953), 649.
{29} F. Hoyle, Annual Review of Astronomy and Astrophysics, 20 (1982): 16.
{30} Stephen Hawking, Physics Bulletin: Cambridge, 32 (1980), 15.
{31} John Barrow and Frank Tepler, The Anthropic Cosmological Principle, 371.
{32} Reinhard Breuer, The Anthropic Principle: Man as the Focal Point of Nature (Boston: Birkhauser, 1990), 102.
{33} John Wheeler, Reader's Digest, September 1986, 107.
{34} Paul Davies, The Accidental Universe (Cambridge: Cambridge University Press, 1982), 90.
{35} Lawrence M. Krauss, "Cosmological Antigravity," Scientific American, 280 (January 1999): 53-59.
{36} F. D. Drake and Dava Sobel, Is Anyone Out There? (New York : Delacorte Press, 1992) 62.
{37} I. S. Shklovskii and C. Sagan, Intelligent Life in the Universe (New York: Dell, 1966).
{38} Robert Rood and James S. Trefil, Are We Alone? The Possibility of Extraterrestrial Civilizations (New York: Scribner, 1981).
{39} Peter B. Ward and Donald Brownlee, Rare Earth: Why Complex Life is Uncommon in the Universe (New York: Copernicus, 2000).
{40} Ibid, 37.
{41} W. Broecker, How to Build a Habitable Planet (Palisades, NY: Eldigio Press, 1985) , 197-229.
{42} Ward and Brownlee, Rare Earth, 19-20.
{43} Ibid, p. 15-33.
{44} J. Kasting, "Habitable Zones Around Stars: An Update," in Circumstellar Habitable Zones, ed. L. Doyle (Menlo Park, CA: Travis House, 1996), 17-28.
{45} Ward and Brownlee, Rare Earth, 208.
{46} Ward and Brownlee, Rare Earth, 264-65.
{47} Walter L. Bradley and Charles B. Thaxton, "Information and the Origin of Life", in The Creation Hypothesis: Scientific Evidence for an Intelligent Designer, ed. J.P. Moreland (Downers Grove, Ill: InterVarsity Press, 1994), 190.
{48} Kenneth R. Miller and Joseph Levine, Biology: The Living Science (Upper Saddle River, New Jersey: Prentice Hall), 1998, p.406-407.
{49} Nocholas Wade, "Genetic Analysis Yields Intimations of a Primordial Commune" (New York: New York Times, June 14th, 2000), from website.
{50} Charles B. Thaxton, Walter L. Bradley, and Roger L. Olsen. Mystery of Life's Origin: Reassessing Current Theories (New York: Philosophical Library, 1984).
{51} Freeman J. Dyson, cited in Barrow and Tipler, Anthropic Cosmological Principle, 318.
{52} Arno Penzias, Our Universe: Accident or Design (Wits 2050, S. Africa :Starwatch, 1992), 42.
{53} Sir Fred Hoyle, The Nature of the Universe (New York: Harper, c1950), 101.
{54} Fred Hoyle, The Intelligent Universe (London: Michael Joseph, 1983), 220
{55} ABC's Nightline with Ted Koppel, April 24, 1992.

Blueprint for a Habitable Universe - Mathematics and the Deep Structure of the Universe 1

Dr. Walter L. Bradley: Is There Scientific Evidence for the Existence of God? How the Recent Discoveries Support a Designed Universe 20 August 2010
Yet even the splendid orderliness of the cosmos, expressible in the mathematical forms, is only a small first step in creating a universe with a suitable place for habitation by complex, conscious life. 

Johannes Kepler, Defundamentis Astrologiae Certioribus, Thesis XX (1601)
"The chief aim of all investigations of the external world should be to discover the rational order and harmony which has been imposed on it by God and which He revealed to us in the language of mathematics."

The particulars of the mathematical forms themselves are also critical. Consider the problem of stability at the atomic and cosmic levels. Both Hamilton's equations for non-relativistic, Newtonian mechanics and Einstein's theory of general relativity are unstable for a sun with planets unless the gravitational potential energy is correctly proportional to, a requirement that is only met for a universe with three spatial dimensions. For Schrödinger's equations for quantum mechanics to give stable, bound energy levels for atomic hydrogen (and by implication, for all atoms), the universe must have no more than three spatial dimensions. Maxwell's equations for electromagnetic energy transmission also require that the universe be no more than three-dimensional. Richard Courant illustrates this felicitous meeting of natural laws with the example of sound and light: "[O]ur actual physical world, in which acoustic or electromagnetic signals are the basis of communication, seems to be singled out among the mathematically conceivable models by intrinsic simplicity and harmony. To summarize, for life to exist, we need an orderly (and by implication, intelligible) universe. Order at many different levels is required. For instance, to have planets that circle their stars, we need Newtonian mechanics operating in a three-dimensional universe. For there to be multiple stable elements of the periodic table to provide a sufficient variety of atomic "building blocks" for life, we need atomic structure to be constrained by the laws of quantum mechanics. We further need the orderliness in chemical reactions that is the consequence of Boltzmann's equation for the second law of thermodynamics. And for an energy source like the sun to transfer its life-giving energy to a habitat like Earth, we require the laws of electromagnetic radiation that Maxwell described.

Our universe is indeed orderly, and in precisely the way necessary for it to serve as a suitable habitat for life. The wonderful internal ordering of the cosmos is matched only by its extraordinary economy. Each one of the fundamental laws of nature is essential to life itself. A universe lacking any of the laws would almost certainly be a universe without life. Many modern scientists, like the mathematicians centuries before them, have been awestruck by the evidence for intelligent design implicit in nature's mathematical harmony and the internal consistency of the laws of nature. 

Paul Davies:  Superforce, page 243
All the evidence so far indicates that many complex structures depend most delicately on the existing form of these laws. It is tempting to believe, therefore, that a complex universe will emerge only if the laws of physics are very close to what they are....The laws, which enable the universe to come into being spontaneously, seem themselves to be the product of exceedingly ingenious design. If physics is the product of design, the universe must have a purpose, and the evidence of modern physics suggests strongly to me that the purpose includes us.
https://3lib.net/book/14357613/6ebdf9

Sir Fred Hoyle:  [Fred Hoyle, in Religion and the Scientists, 1959; quoted in Barrow and Tipler, p. 22]
I do not believe that any scientist who examines the evidence would fail to draw the inference that the laws of nuclear physics have been deliberately designed with regard to the consequences they produce inside stars. If this is so, then my apparently random quirks have become part of a deep-laid scheme. If not then we are back again at a monstrous sequence of accidents.

Nobel laureates Eugene Wigner and Albert Einstein have respectfully evoked "mystery" or "eternal mystery" in their meditations upon the brilliant mathematical encoding of nature's deep structures. But as Kepler, Newton, Galileo, Copernicus, Davies, and Hoyle and many others have noted, the mysterious coherency of the mathematical forms underlying the cosmos is solved if we recognize these forms to be the creative intentionality of an intelligent creator who has purposefully designed our cosmos as an ideal habitat for us.

Blueprint for a Habitable Universe: Universal Constants - Cosmic Coincidences?

Next, let us turn to the deepest level of cosmic harmony and coherence - that of the elemental forces and universal constants which govern all of nature. Much of the essential design of our universe is embodied in the scaling of the various forces, such as gravity and electromagnetism, and the sizing of the rest mass of the various elemental particles such as electrons, protons, and neutrons.
There are certain universal constants that are indispensable for our mathematical description of the universe. These include Planck's constant, h; the speed of light, c; the gravity-force constant, G; the rest masses of the proton, electron, and neutron; the unit charge for the electron or proton; the weak force, strong nuclear force, electromagnetic coupling constants; and Boltzmann's constant, k.

When cosmological models were first developed in the mid-twentieth century, cosmologists naively assumed that the selection of a given set of constants was not critical to the formation of a suitable habitat for life. Through subsequent parametric studies that varied those constants, scientists now know that relatively small changes in any of the constants produce a dramatically different universe and one that is not hospitable to life of any imaginable type.

Twentieth-century physicists have identified four fundamental forces in nature. These may each be expressed as dimensionless numbers to allow a comparison of their relative strengths. These values vary by a factor of 10⁴¹ (10 with forty additional zeros after it), or by 41 orders of magnitude. Yet modest changes in the relative strengths of any of these forces and their associated constants would produce dramatic changes in the universe, rendering it unsuitable for life of any imaginable type. Several examples to illustrate this fine-tuning of our universe are presented next.

Balancing Gravity and Electromagnetism Forces - Fine Tuning Our Star and Its Radiation
The electromagnetic force is 10^³⁸ times stronger than the gravity force. Gravity draws hydrogen into stars, creating a high-temperature plasma. The protons in the plasma must overcome their electromagnetic repulsion to fuse. Thus the relative strength of the gravity force to the electromagnetic force determines the rate at which stars "burn" by fusion. If this ratio of strengths were altered to 10^³² instead of 10^³⁸ (i.e., if gravity were much stronger), stars would be a billion times less massive and would burn a million times faster.

Electromagnetic radiation and the light spectrum also depend on the relative strengths of the gravity and electromagnetic forces and their associated constants. Furthermore, the frequency distribution of electromagnetic radiation produced by the sun must be precisely tuned to the energies of the various chemical bonds on Earth. Excessively energetic photons of radiation (i.e., the ultraviolet radiation emitted from a blue giant star) destroy chemical bonds and destabilize organic molecules. Insufficiently energetic photons (e.g., infrared and longer wavelength radiation from a red dwarf star) would result in chemical reactions that are either too sluggish or would not occur at all. All life on Earth depends upon fine-tuned solar radiation, which requires, in turn, a very precise balancing of the electromagnetic and gravitational forces.
As previously noted, the chemical bonding energy relies upon quantum mechanical calculations that include the electromagnetic force, the mass of the electron, the speed of light (c), and Planck's constant (h). Matching the radiation from the sun to the chemical bonding energy requires that the magnitude of six constants be selected to satisfy the following inequality, with the caveat that the two sides of the inequality are of the same order of magnitude, guaranteeing that the photons are sufficiently energetic, but not too energetic.

In what is either an amazing coincidence or careful design by an intelligent Creator, these constants have the very precise values relative to each other that are necessary to give a universe in which radiation from the sun is tuned to the necessary chemical reactions that are essential for life. The greatest intensity of radiation from the sun occurs at the place of greatest biological utility. It is uniquely this same wavelength of radiation that is idea to foster the chemistry of life. This is either a truly amazing series of coincidences or else the result of careful design.

Happily, our star (the sun) emits radiation (light) that is finely tuned to drive the chemical reactions necessary for life. But there is still a critical potential problem: getting that radiation from the sun to the place where the chemical reactions occur. Passing through the near vacuum of space is no problem. However, absorption of light by either Earth's atmosphere or by water where the necessary chemical reactions occur could render life on Earth impossible. It is remarkable that both the Earth's atmosphere and water have "optical windows" that allow visible light (just the radiation necessary for life) to pass through with very little absorption, whereas shorter wavelength (destructive ultraviolet radiation) and longer wavelength (infrared) radiation are both highly absorbed.  This allows solar energy in the form of light to reach the reacting chemicals in the universal solvent, which is water.

Considering the importance of visible sunlight for all aspects of terrestrial life, one cannot help being awed by the dramatically narrow window in the atmospheric absorption...and in the absorption spectrum of water.
It is remarkable that the optical properties of water and our atmosphere, the chemical bonding energies of the chemicals of life, and the radiation from the sun are all precisely harmonized to allow living systems to utilize the energy from the sun, without which life could not exist. It is quite analogous to your car, which can only run using gasoline as a fuel. Happily, but not accidentally, the service station has an ample supply of exactly the right fuel for your automobile. But someone had to drill for and produce the oil, someone had to refine it into liquid fuel (gasoline) that has been carefully optimized for your internal combustion engine, and others had to truck it to your service station. The production and transportation of the right energy from the sun for the metabolic motors of plants and animals is much more remarkable, and hardly accidental.

Finally, without this unique window of light transmission through water, which is constructed upon an intricate framework of universal constants, vision would be impossible and sight-communication would cease, since living tissue and eyes are composed mainly of water.

Nuclear Strong Force and Electromagnetic Force - Finely Balanced for a Universe Rich in Carbon and Oxygen (and therefore water)
The nuclear strong force is the strongest force within nature, occurring at the subatomic level to bind protons and neutrons within atomic nuclei.{25} Were we to increase the ratio of the strong force to the electromagnetic force by only 3.4 percent, the result would be a universe with no hydrogen, no long-lived stars that burn hydrogen, and no water (a molecule composed of two hydrogen atoms and one oxygen atom)--our "universal solvent" for life. Likewise, a decrease of only 9 percent in the strong force relative to the electromagnetic force would decimate the periodic table of elements. Such a change would prevent deuterons from forming from the combination of protons and neutrons. Deuterons, in turn, combine to form helium, then helium fuses to produce beryllium, and so forth.{26}
Within the nucleus, an even more precise balancing of the strong force and the electromagnetic force allows for a universe with an abundance of organic building blocks, including both carbon and oxygen.{27} Carbon serves as the universal connector for organic life and is an optimal reactant with almost every other element, forming bonds that are stable but not too stable, allowing compounds to be formed and disassembled. Oxygen is a component of water, the necessary universal solvent where life chemistry can occur. This is why when people speculate about life on Mars, they first look for signs of organic molecules (ones containing carbon) and signs that Mars once had water.
Quantum physics examines the most minute energy exchanges at the deepest levels of the cosmic order. Only certain energy levels are permitted within nuclei-like steps on a ladder. If the mass-energy for two colliding particles results in a combined mass-energy that is equal to or slightly less than a permissible energy level on the quantum "energy ladder," then the two nuclei will readily stick together or fuse on collision, with the energy difference needed to reach the step being supplied by the kinetic energy of the colliding particles. If this mass-energy level for the combined particles is exactly right, then the collisions are said to have resonance, which is to say that there is a high efficiency within the collision. On the other hand, if the combined mass-energy results in a value that is slightly higher than one of the permissible energy levels on the energy ladder, then the particles will simply bounce off each other rather than fusing, or sticking together.
It is clear that the step sizes between quantum nuclear energy levels depends on the balance between the strong force and the electromagnetic force, and these steps must be tuned to the mass-energy levels of various nuclei for resonance to occur and give an efficient conversion by fusion of lighter element into carbon, oxygen and heavier elements.
In 1953, Sir Fred Hoyle et al. predicted the existence of the unknown resonance energy level for carbon, and it was subsequently confirmed through experimentation.{28} In 1982, Hoyle offered a very insightful summary of the significance he attached to his remarkable predictions.

From 1953 onward, Willy Fowler and I have always been intrigued by the remarkable relation of the 7.65 MeV energy level in the nucleus of 12 C to the 7.12 MeV level in 16 O. If you wanted to produce carbon and oxygen in roughly equal quantities by stellar nucleosynthesis, these are the two levels you would have to fix, and your fixing would have to be just where these levels are actually found to be. Another put-up job? Following the above argument, I am inclined to think so. A common sense interpretation of the facts suggests that a super intellect has "monkeyed" with the physics as well as the chemistry and biology, and there are no blind forces worth speaking about in nature.{29}

The Rest Mass of Subatomic Particles - Key to Universe Rich in Elemental Diversity
Scientists have been surprised to discover the extraordinary tuning of the masses of the elementary particles to each other and to the forces in nature. Stephen Hawking has noted that the difference in the rest mass of the neutron and the rest mass of the proton must be approximately equal to twice the mass of the electron. The mass-energy of the proton is 938.28 MeV and the mass-energy of the neutron is 939.57 MeV. The mass-energy of the electron is 0.51 MeV, or approximately half of the difference in neutron and proton mass-energies, just as Hawking indicated it must be.{30} If the mass-energy of the proton plus the mass-energy of the electron were not slightly smaller than the mass-energy of the neutron, then electrons would combine with protons to form neutrons, with all atomic structure collapsing, leaving an inhospitable world composed only of neutrons.
On the other hand, if this difference were larger, then neutrons would all decay into protons and electrons, leaving a world of pure hydrogen, since neutrons are necessary for protons to combine to build heavier nuclei and the associated elements. As things stand, the neutron is just heavy enough to ensure that the Big Bang would yield one neutron to every seven protons, allowing for an abundant supply of hydrogen for star fuel and enough neutrons to build up the heavier elements in the universe.{31} Again, a meticulous inner design assures a universe with long-term sources of energy and elemental diversity.
The Nuclear Weak Coupling Force - Tuned to Give an Ideal Balance Between Hydrogen (as Fuel for Sun) and Heavier Elements as Building Blocks for Life
The weak force governs certain interactions at the subatomic or nuclear level. If the weak force coupling constant were slightly larger, neutrons would decay more rapidly, reducing the production of deuterons, and thus of helium and elements with heavier nuclei. On the other hand, if the weak force coupling constant were slightly weaker, the Big Bang would have burned almost all of the hydrogen into helium, with the ultimate outcome being a universe with little or no hydrogen and many heavier elements instead. This would leave no long-lived stars and no hydrogen-containing compounds, especially water. In 1991, Breuer noted that the appropriate mix of hydrogen and helium to provide hydrogen-containing compounds, long-term stars, and heavier elements is approximately 75 percent hydrogen and 25 percent helium, which is just what we find in our universe.{32}
This is obviously only an illustrative--but not exhaustive--list of cosmic "coincidences." Clearly, the four forces in nature and the universal constants must be very carefully calibrated or scaled to provide a universe that satisfies the key requirements for life that we enumerated in our initial "needs statement": for example, elemental diversity, an abundance of oxygen and carbon, and a long-term energy source (our sun) that is precisely matched to the bonding strength of organic molecules, with minimal absorption by water or Earth's terrestrial atmosphere.
John Wheeler, formerly Professor of Physics at Princeton, in discussing these observations asks:

Is man an unimportant bit of dust on an unimportant planet in an unimportant galaxy somewhere in the vastness of space? No! The necessity to produce life lies at the center of the universe's whole machinery and design.....Slight variations in physical laws such as gravity or electromagnetism would make life impossible.{33}

Blueprint for a Habitable Universe: The Criticality of Initial or Boundary Conditions

As we already suggested, correct mathematical forms and exactly the right values for them are necessary but not sufficient to guarantee a suitable habitat for complex, conscious life. For all of the mathematical elegance and inner attunement of the cosmos, life still would not have occurred had not certain initial conditions been properly set at certain critical points in the formation of the universe and Earth. Let us briefly consider the initial conditions for the Big Bang, the design of our terrestrial "Garden of Eden," and the staggering informational requirements for the origin and development of the first living system.
The Big Bang
The "Big Bang" follows the physics of any explosion, though on an inconceivably large scale. The critical boundary condition for the Big Bang is its initial velocity. If this velocity is too fast, the matter in the universe expands too quickly and never coalesces into planets, stars, and galaxies. If the initial velocity is too slow, the universe expands only for a short time and then quickly collapses under the influence of gravity. Well-accepted cosmological models{34} tell us that the initial velocity must be specified to a precision of 1/10⁶⁰. This requirement seems to overwhelm chance and has been the impetus for creative alternatives, most recently the new inflationary model of the Big Bang.
Even this newer model requires a high level of fine-tuning for it to have occurred at all and to have yielded irregularities that are neither too small nor too large for the formation of galaxies. Astrophysicists originally estimated that two components of an expansion-driving cosmological constant must cancel each other with an accuracy of better than 1 part in 10⁵⁰. In the January 1999 issue of Scientific American, the required accuracy was sharpened to the phenomenal exactitude of 1 part in 10¹²³.{35} Furthermore, the ratio of the gravitational energy to the kinetic energy must be equal to 1.00000 with a variation of less than 1 part in 100,000. While such estimates are being actively researched at the moment and may change over time, all possible models of the Big Bang will contain boundary conditions of a remarkably specific nature that cannot simply be described away as "fortuitous".
The Uniqueness of our "Garden of Eden"
Astronomers F. D. Drake{36} and Carl Sagan{37} speculated during the 1960s and 1970s that Earth-like places in the universe were abundant, at least one thousand but possibly as many as one hundred million. This optimism in the ubiquity of life downplayed the specialness of planet Earth. By the 1980s, University of Virginia astronomers Trefil and Rood offered a more sober assessment in their book, Are We Alone? The Possibility of Extraterrestrial Civilizations.{38} They concluded that it is improbable that life exists anywhere else in the universe. More recently, Peter Douglas Ward and Donald Brownlee of the University of Washington have taken the idea of the Earth's unique place in our vast universe to a much higher level. In their recent blockbuster book, Rare Earth: Why Complex Life is Uncommon in the Universe,{39} they argue that the more we learn about Earth, the more we realize how improbable is its existence as a uniquely habitable place in our universe. Ward and Brownlee state it well:

If some god-like being could be given the opportunity to plan a sequence of events with the expressed goal of duplicating our 'Garden of Eden', that power would face a formidable task. With the best of intentions but limited by natural laws and materials it is unlikely that Earth could ever be truly replicated. Too many processes in its formation involve sheer luck. Earth-like planets could certainly be made, but each would differ in critical ways. This is well illustrated by the fantastic variety of planets and satellites (moons) that formed in our solar system. They all started with similar building materials, but the final products are vastly different from each other . . . . The physical events that led to the formation and evolution of the physical Earth required an intricate set of nearly irreproducible circumstances.{40}

What are these remarkable coincidences that have precipitated the emerging recognition of the uniqueness of Earth? Let us consider just two representative examples, temperature control and plate tectonics, both of which we have alluded to in our "needs statement" for a habitat for complex life.
Temperature Control on Planet Earth
In a universe where water is the primary medium for the chemistry of life, the temperature must be maintained between 0° C and 100° C (32° F to 212° F) for at least some portion of the year. If the temperature on earth were ever to stay below 0° C for an extended period of time, the conversion of all of Earth's water to ice would be an irreversible step. Because ice has a very high reflectivity for sunlight, if the Earth ever becomes an ice ball, there is no returning to the higher temperatures where water exists and life can flourish. If the temperature on Earth were to exceed 100°C for an extended period of time, all oceans would evaporate, creating a vapor canopy. Again, such a step would be irreversible, since this much water in the atmosphere would efficiently trap all of the radiant heat from the sun in a "super-greenhouse effect," preventing the cooling that would be necessary to allow the steam to re-condense to water.{41} This appears to be what happened on Venus.
Complex, conscious life requires an even more narrow temperature range of approximately 5-50° C.{42} How does our portion of real estate in the universe remain within such a narrow temperature range, given that almost every other place in the universe is either much hotter or much colder than planet Earth, and well outside the allowable range for life? First, we need to be at the right distance from the sun. In our solar system, there is a very narrow range that might permit such a temperature range to be sustained, as seen in Fig. 1. Mercury and Venus are too close to the sun, and Mars is too far away. Earth must be within approximately 10% of its actual orbit to maintain a suitable temperature range.{43}
Yet Earth's correct orbital distance from the sun is not the whole story. Our moon has an average temperature of -18° C, while Earth has an average temperature of 33° C; yet each is approximately the same average distance from the sun. Earth's atmosphere, however, efficiently traps the sun's radiant heat, maintaining the proper planetary temperature range. Humans also require an atmosphere with exactly the right proportion of tri-atomic molecules, or gases like carbon dioxide and water vapor. Small temperature variations from day to night make Earth more readily habitable. By contrast, the moon takes twenty-nine days to effectively rotate one whole period with respect to the sun, giving much larger temperature fluctuations from day to night. Earth's rotational rate is ideal to maintain our temperature within a narrow range.


https://web.archive.org/web/20110805203154/http://www.leaderu.com/real/ri9403/evidence.html

Honeycomb's hexagon, by evolution, or design?

https://reasonandscience.catsboard.com/t1360-evidence-of-design-in-mathematics#9071

Around 36 B.C., Marcus Terentius Varro, in his book on agriculture, wrote about the hexagonal form of the bee’s honeycomb. Varro wrote, “Does not the chamber in the comb have six angles . . . The geometricians prove that this hexagon inscribed in a circular figure encloses the greatest amount of space. If the cells are not contiguous foreign matter could enter the interstices between them and so defile the purity of their produce". 1

In a 2019 interview, Thomas Hales—the mathematician who finally proved the conjecture—said that ultimately, “A hexagonal honeycomb is the way to fit the most area with the least perimeter.” From a bee’s perspective, that means storing more honey in a larger volume while spending less energy building a structure to contain it.

Then, the author adds: In other words, Darwin was right.

Charles Darwin wrote: He must be a dull man who can examine the exquisite structure of a comb, so beautifully adapted to its end, without enthusiastic admiration.

My comment: Darwin would be right if his theory explained the origin of the structure adopted by Bees.

David F. Coppedge: Do Honeycombs Just Happen, or Do Bees Design Them? July 19, 2013

Live Science stated that the honeycomb, “once thought to be an incredible feat of math-savvy insects” has been “explained by simple mechanics.”  Later in that article, though, is the suggestion that bees focus their body heat to shape the hexagonal cells after first carving them out as cylinders.  Another biologist spoke of the “the mechanisms that honeybees manage to build very precise cells,” suggesting there is more going on than “simple mechanics.”  That biologist also hinted that humans could learn something from the bees’ techniques.


If honeycombs were the product of blind physics alone, why are they so precise in beehives?  Columnar basalt is an example of natural law at work without design.  When some lava flows cool, they crack into polygonal shapes, usually hexagons—but not always.  Displays like Devil’s Postpile in California, spectacular as they are, show the limits of natural law; irregular polygons, falling into piles at the base.  Nothing forces them to assemble at precise angles or thicknesses for any conceivable function.  Similarly, bubbles on the surface of water can sometimes assume hexagonal borders due to surface tension, but are rarely free of defects.  Honeycomb hexagons, by contrast, are very orderly and regular, maximizing space and minimizing wax, for a specified purpose: creating space for honey storage and the raising of young.
Another example can be found with arches.  Natural arches can be very large and spectacular, but we can tell intuitively whether an arch is natural or designed.  The Arc de Triomphe in Paris, the arches in a basement supporting a building, or the arches in a Roman aqueduct spanning a canyon for miles, would never result from natural law.  Why do they differ from Delicate Arch in Utah?  Delicate Arch doesn’t do anything.  It has no specification, no purpose.  There, a sandstone fin eroded, weakest part first, till the most stable structure – an arch – formed and enlarged till it stands near to collapse, joining other arches in the park where gravity took over.  No mind was involved.  The man-made arches, though, required a mind. They function for artistry (commemorating a military victory), for architecture, or for carrying water.  Because they function, the design specs for them are more critical and precise.  Some Roman aqueducts, still standing today, maintained a very, very slight declination to keep the water flowing for over 30 miles, despite hills and canyons along the route.
Just because bees know how to use surface tension does not mean they are bystanders in a blind process of physics.  On the contrary, knowing how to use natural law efficiently is evidence of intelligent design.  If a bee can start with a round hole and use surface tension to help mold it into a hexagon, the bee is working smart, just as much as an engineer using gravity to advantage.  The bee doesn’t just let nature do it.  The bee supervises the result, ensuring that the resulting honeycomb meets the requirements for precise wall thicknesses and inclinations of the cells.


The intelligent design in the case of honeycomb construction resides not in the brains of the bees themselves, but in the instinctive abilities programmed into them.  They carry out the programmed instincts like miniature robots.  That presupposes a robot-maker.  Who was it?  That’s an interesting question, but it’s beyond the scope of intelligent design theory.  Just as one can tell an aqueduct was designed without knowing the designer, one can infer intelligent design in honeycombs from the specified complexity observed, whether or not certain natural laws come into play during its construction.  The observation of design does not require knowing the identify of the designer, but makes belief in a personal, purposeful God, such as the God of the Bible, the most reasonable step of faith in the direction the evidence points.

JOURNAL ARTICLE Bioinspired engineering of honeycomb structure – Using nature to inspire human innovation
“Through thousands of years of exploration, we have gone beyond the traditional awareness of the exceptionally high mechanical strength as the only characteristic of honeycomb structures, and have gradually deepened our understanding of multifunctional design principles for honeycomb structures.” 3

Thomas C. Hales: The Honeycomb Conjecture Discrete Computational Geometry |
“In part because of the isoperimetric property of the honeycomb, there is a vast literature through the centuries mentioning the bee as a geometer. . . During the 18th century, the mathematical architecture of the honeycomb was viewed as evidence of a great teleological tendency of the universe.”

1. https://arxiv.org/pdf/math/9906042.pdf
2. https://asknature.org/strategy/honeycomb-structure-is-space-efficient-and-strong/
3. https://www.sciencedirect.com/science/article/abs/pii/S0079642515000377

Dr. Joshua M. Moritz: Math and the Mind of God August 2, 2022

ccording to the German mathematician Georg Cantor, there are three levels of existence: the physical universe, the human mind, and the Mind of God.

These days we are quite comfortable with the notion of infinity. As scientists discuss the possibility of an infinite multiverse, our entertainment features infinity wars and superheroes who travel to infinity and beyond. Yet, in the past, infinity was not so easy to come by. This is because whenever the greatest thinkers in history pondered the idea of infinity, they soon realized that this concept is riddled with paradox, breaking all the rules of common sense. Logically speaking, infinity didn’t add up.

The Paradox of Infinity
Take, for example, the Achilles Paradox articulated by the ancient Greek philosopher Zeno. If the space or distance between point A and point B can be divided into increasingly smaller segments, then there is no such thing as the smallest distance since there can always be one that is smaller. There are thus an infinite number of smaller segments between point A and point B. But this means that if the Greek hero Achilles wants to run from point A to point B, it would take him an eternity to reach his destination because he has an infinite number of small spaces to traverse. And yet somehow Achilles can move from point A to point B.

Such paradoxes and contradictions led another Ancient Greek philosopher, Aristotle, to distinguish two different types of infinites. The first is an actual infinite, an infinite that embraces everything in reality, leaving nothing outside.

The second is a potential infinite, which Aristotle describes as a type of infinity that always has something outside of it. In other words, a potential infinite is a situation where you can always add one more number. As Aristotle says, “one thing is always being taken after another, and each thing that is taken is always finite, but always different.”1 However large a finite number you have reached, you can potentially add more, but you can never actually have all of the numbers as a completed set or group. Because you can never actually count to the highest number, Aristotle declared the existence of an actual infinite to be philosophically forbidden.

Like his teacher Plato, Aristotle believed that the actual world was logically constructed and not a realm of contradiction and chaos. Consequently, he believed that there are no actual infinities in physical reality.

From Infinity to God
Still, one actual infinity lurked at the heart of Aristotle’s physics that went unnoticed for almost a thousand years—his idea that the physical world was eternal. The contradiction at the core of Aristotle’s philosophy was first pointed out by John Philoponus, an Alexandrian mathematician, philosopher, scientist, and Christian theologian. Philoponus demonstrated that if the cosmos is uncreated and has no beginning (as Aristotle argued), then an actual infinity of years must have passed. If the world is eternal, then an infinite number of moments must have been traversed. However, if the infinite has been traversed, then it is not truly the infinite. Philoponus used this paradox of infinity to prove that a transcendent God must have created the universe. Philosopher and historian Richard Sorabji explains that Philoponus “found a contradiction at the heart of paganism, a contradiction between their concept of infinity and their denial of a beginning,” and this was a key “turning point in the history of philosophy.”

The Mathematical Mysteries of Infinity
More than a thousand years after Philoponus, Galileo continued to explore the mysteries of infinity through mathematics. In 1638 Galileo discovered a paradox of infinity as it concerned the set of natural numbers (1, 2, 3, 4, 5…) compared to their squares (1, 4, 9, 16, 25…). A common sense approach would lead one to believe that there are more natural numbers (N) than squares (N2) because not every natural number is a square. However, Galileo set up a one-to-one correspondence between these sets to show that the number of the elements of N is equal to the number of the elements of N2—because there is always a next highest number. In other words, these two sets become equal when infinity is brought into the picture (since they are both infinite). Galileo’s paradox reveals that infinite collections don’t obey the rules of common sense. Even if one subtracts an infinity from an infinity, one still has an infinity left over. Infinity appears to give us something for free without using anything up.

From God to Infinity
It took the genius of Georg Cantor to show how to make sense of the paradoxes of infinity that Galileo had first identified three hundred years before. Cantor founded set theory, discovering that some infinities are bigger than others. Through these discoveries, explains mathematical physicist John Barrow, “Cantor produced a theory that answered all the objections of his predecessors and revealed the unexpected richness hiding in the realm of the infinite.”3 Cantor’s set theory was a revolution in the history of mathematics because it overthrew all the assumptions that previous generations held about infinity.

Today, says mathematician Ian Stewart, “the fruits of Cantor’s labors form the basis of the whole of mathematics.”4 According to Cantor himself, his insights into the nature of mathematics and infinity were revealed to him by God. As he states in a letter from 1883: “I am far from claiming my discoveries are due to personal merit, because I am only an instrument of a Higher Power that will continue to work long after me, just as it revealed itself thousands of years ago to Euclid and Archimedes.”

To demonstrate how some infinities are larger than others, Cantor introduced the mathematical concept of a “completed set.” For example, Cantor considered the natural numbers (N = 1, 2, 3, 4…) together as a set in themselves, as a completed infinite magnitude. Taken as a whole, Cantor defined the set of natural numbers as the first “transfinite number” (denoted by the lowercase omega, ω), and he deliberately distinguished ω from the infinity symbol, ∞, which had been introduced by John Wallis in 1655 to mean simply “unbounded.”

Next, Cantor defined a countable infinity to be one that can be put into one-to-one correspondence with the list of natural numbers (1, 2, 3, 4, 5, 6, . . .). Thus, for example, the even numbers are countably infinite, and so are all the odd numbers. All countably infinite sets have the same ‘size’ in Cantor’s sense. Cantor understood these to be the smallest infinities that could exist and denoted them by the first letter of the Hebrew alphabet, the symbol Aleph-nought (ℵ0). He revealed that all the fractions formed by dividing one whole number by another are also countably infinite. All the infinities that philosophers and mathematicians discussed in ancient times were countable infinities in Cantor’s sense.

Cantor then set about demonstrating that an unending catalog of mathematical infinities exists. He showed how precise definitions of things like infinite sets lead to the conclusion that ever larger ones can be defined. In his discussion of mathematical reality, Cantor distinguished three levels of existence: (1) the mind of God, (2) the human mind, and (3) the physical universe. “The set of everything” and “the set of all sets” belonged to Absolute Infinity, which is beyond mathematical formulation and which is comprehended only in the Mind of God. Cantor believed that God put the concept of number, both finite and transfinite, into the human mind and that their existence in the Mind of God was the basis for their existence in the human mind. He differentiated between the transfinite numbers, as created in the physical universe and in the human mind, and eternal and uncreated Absolute Infinity, which was reserved for God and his attributes.

While infinites existed mathematically, Cantor did not believe that the universe was infinite in either duration or extent. Cantor firmly believed that God had created the universe in the beginning. The only actual infinity is God. Citing Augustine’s City of God, Cantor affirmed: “All infinity is in some ineffable way made finite to God, for it is comprehended by his knowledge.”6 For Cantor, then, God’s infinity is both the beginning and the end—the alpha and the omega—of all other infinities.

Aristotle, Physics, 3.6.206a.27-29.
Richard Sorabji, Philoponus and the Rejection of Aristotelian Science (Cornell University Press: 1987) 220.
John D. Barrow, The Infinite Book: A Short Guide to the Boundless, Timeless and Endless (Knopf Doubleday , 2007), 67.
Ian Stewart, From Here to Infinity (Oxford University Press, 1996) 67
Georg Cantor, Letter from Cantor to Professor C.A. Valson (Halle January 31, 1886)
Augustine, City of God, 2.238

https://aish.com/math-and-the-mind-of-god/

Evidence of Design in Mathematics

Mathematics can be thought of as a creative endeavor where mathematicians establish the rules and explore the consequences within those frameworks. In contrast, physics operates within a realm where the rules are not a matter of choice but are dictated by the very fabric of the universe. It's fascinating to observe that the mathematical structures devised by human intellect often align with the principles governing the physical world. This alignment raises questions about the origin of nature's laws. Why do the abstract concepts and models developed in the realm of mathematics so accurately describe the workings of the physical universe? This congruence suggests that mathematics and physics are intertwined, with mathematics providing the language and framework through which we understand physical reality. One might consider physics as the expression of mathematical principles in the tangible world, where matter and energy interact according to these underlying rules. This perspective positions mathematics not merely as a tool for describing physical phenomena but as a fundamental aspect of the universe's structure. The natural world, in all its complexity, seems to operate according to a set of mathematical principles that exist independently of human thought.

The question then becomes: What is the source of these mathematical rules that nature so faithfully adheres to? Are they inherent in the cosmos, an intrinsic part of the universe's fabric, or are they a product of the human mind's attempt to impose order on the chaos of existence? This inquiry touches upon philosophical and metaphysical realms, pondering whether the universe is inherently mathematical or if our understanding of it as such is a reflection of our cognitive frameworks. The remarkable effectiveness of mathematics in describing the physical world hints at a deeper order, suggesting that the universe might be structured in a way that is inherently understandable through mathematics. This notion implies that the mathematical laws we uncover through exploration and invention may reflect a more profound cosmic order, where the principles governing the universe resonate with the mathematical constructs conceived by the human mind. Thus, the exploration of physics and mathematics becomes a journey not just through the external world but also an introspective quest, seeking to understand the very nature of reality and our place within it. It invites us to consider the possibility that the universe is not just described by mathematics but is fundamentally mathematical, with its deepest truths encoded in the language of numbers and equations.

The mathematical foundation of the universe

The concept that "the universe is mathematical" proposed by Max Tegmark, though intriguing, raises questions about categorizing the universe, which is inherently physical, as fundamentally mathematical. This leads to a consideration that perhaps the mathematical laws governing the universe originated in the mind of a creator, a divine intellect, and were implemented in the act of creation. This perspective invites a reflection on the human capacity to grasp and apply the abstract realm of mathematics to understand the universe, hinting at a deeper connection or correspondence between human cognition and the cosmic order. It suggests that the remarkable ability of humans to decipher the universe's workings through mathematics might reflect a shared origin or essence with the very fabric of the cosmos, possibly pointing to a creator who endowed the universe with mathematical order and gifted humans with the ability to perceive and understand it.

This is a concept that has been discovered by many mathematicians, who often feel they are not so much inventing mathematical structures as uncovering them. This suggests that these structures have an existence independent of human thought. The universe presents itself as a deeply mathematical and geometrically structured entity, displaying a level of organization and harmony that is hard for the human mind to overlook. This system, inherent in the fabric of the cosmos, points to an underlying mathematical order. Many in the fields of mathematics and physics hold the view that the realm of mathematical concepts exists independently of the physical universe, within a timeless and spaceless domain of abstract ideas. Max Tegmark, a prominent voice in this discussion, asserts that mathematical structures are not invented but discovered by humans, who merely devise the notations to describe these pre-existing entities. This suggests that these structures reside in an abstract domain, accessible to mathematicians and physicists through rigorous intellectual effort, allowing them to draw parallels between these abstract concepts and the physical phenomena observed in the world. Remarkably, despite the individual and subjective nature of these intellectual endeavors, physicists worldwide often converge on a unified understanding of these laws, a consensus rarely achieved outside the realm of physical sciences. To truly grasp the fundamental nature of reality, one must look beyond linguistic constructs to the mathematics itself, implying that the ultimate nature of external reality is intrinsically mathematical. The existence of these mathematical truths, predating human consciousness, suggests that mathematics itself is the foundational reality upon which the universe is built.


While the physicist Max Tegmark boldly claims that mathematics is not merely a descriptive language but the very fabric of existence itself, it might be more case-adequate to argue that this mathematical underpinning points to an even deeper source – the workings of a conscious, intelligent designer. Rather than mathematics operating in a "god-like" fashion, as Tegmark suggests, the beautiful and coherent mathematical laws governing our universe are themselves the product of a supreme creative mind – God. In this view, the three fundamental ingredients that makeup reality are: 1) a conscious, intelligent source (God), 2) the abstract language of mathematics as the blueprint, and 3) the material world as the manifestation of that blueprint. Just as our own nonphysical thoughts inexplicably guide the actions of our physical bodies, we can draw a parallel to how the nonphysical realm, God, uses mathematics to dictate the behavior and workings of the physical universe. This mysterious connection between the abstract and the concrete is evidence of intelligent design that transcends our current scientific understanding. Rather than mathematics being the ultimate, self-existing reality, it is more plausible that this profound mathematical language is the carefully crafted creation of supreme intelligence – an expression of divine wisdom and creativity. In this framework, the elegance and coherence of the mathematical laws that permeate our universe are not mere happenstance but a testament to the genius of a transcendent designer.

The order of the cosmos, represented through various mathematical laws, is merely the foundation for a universe capable of supporting complex, conscious life. The specific nature of these mathematical laws is crucial for stability at both atomic and cosmic scales. For example, the stability of solar systems and the formation of stable, bound energy levels within atoms both hinge on the universe being three-dimensional. Similarly, the transmission of electromagnetic energy, crucial for phenomena like light and sound, is contingent upon this three-dimensionality.

This remarkable alignment of natural laws underpins the possibility of communication through sound and light in our physical reality, highlighting a universe distinguished by its inherent simplicity and harmony among conceivable mathematical models. To facilitate life, an orderly universe is necessary, extending from the macroscopic stability of planetary orbits to the microscopic stability of atomic structures. Newtonian mechanics, quantum mechanics, and thermodynamic principles, along with electromagnetic laws, all contribute to an environment where life as we know it can flourish. These laws ensure the existence of diverse atomic "building blocks," govern chemical interactions, and enable the sun to nourish life on planets like Earth. The universe's orderliness, essential for life, showcases the extraordinary interplay and necessity of fundamental natural laws. The absence of any of these laws could render the universe lifeless. This profound mathematical harmony and the coherence of natural laws have led many scientists to marvel at the apparent intelligent design within the universe's fabric.

Sir Fred Hoyle, a distinguished British astronomer, remarked on the design of nuclear physics laws as observed within stellar phenomena, suggesting that any scientist who deeply considers this evidence might conclude these laws have been intentionally crafted to yield the observed outcomes within stars. This notion posits that what may seem like random quirks of the universe could actually be components of a meticulously planned scheme; otherwise, we are left with the improbable likelihood of a series of fortunate coincidences. Nobel laureates such as Eugene Wigner and Albert Einstein have invoked the concept of "mystery" or "eternal mystery" when reflecting on the precise mathematical formulation of nature's underlying principles. This sentiment is echoed by luminaries like Kepler, Newton, Galileo, Copernicus, Paul Davies, and Hoyle, who have suggested that the coherent mathematical structure of the cosmos can be understood as the manifestation of an intelligent creator's deliberate intention, designed to make our universe a conducive habitat for life. In exploring the essence of cosmic harmony, attention turns to the elemental forces and universal constants that govern the entirety of nature. The foundational architecture of our universe is encapsulated in the relationships between forces such as gravity and electromagnetism, and the defined rest masses of elementary particles like electrons, protons, and neutrons.

Key universal constants essential for the mathematical depiction of the universe include Planck's constant (h), the speed of light (c), the gravitational constant (G), the rest masses of the proton, electron, and neutron, the elementary charge, and the constants associated with the weak and strong nuclear forces, electromagnetic coupling, and Boltzmann's constant (k). These constants and forces are integral to the balanced design that allows our universe to exist in a state that can support life. In the initial stages of developing cosmological models during the mid-20th century, cosmologists had the simplistic assumption that the choice of universal constants was not particularly crucial for creating a universe capable of supporting life. However, detailed studies that experimented with altering these constants have revealed that even minor adjustments could lead to a universe vastly different from ours, one incapable of supporting any conceivable form of life. The fine-tuned nature of our universe has captured the imagination of both the scientific community and the public, inspiring a wide array of publications exploring this theme, such as discussions on the Anthropic Cosmological Principle, the notion of an Accidental Universe, and various explorations into Cosmic Coincidences and the idea of Intelligent Design.

Albert Einstein famously remarked, "As far as the laws of mathematics refer to reality, they are not certain; and as far as they are certain, they do not refer to reality." This statement captures the intriguing paradox at the heart of physics and mathematics. On one hand, mathematics possesses a level of certainty and precision unmatched by any other intellectual endeavor, owing to its logical structure and self-contained proofs. On the other hand, when we apply mathematical concepts to the physical world, a degree of uncertainty emerges, as the complexities and variabilities of reality do not always conform neatly to mathematical ideals. Einstein's observation invites us to ponder the relationship between the abstract world of mathematics and the tangible reality of physics. Mathematics, with its elegant theorems and rigorous proofs, offers a level of certainty that derives from its logical foundations. However, this certainty is confined to the realm of mathematical constructs, independent of the empirical world. When we attempt to map these constructs onto physical phenomena, the unpredictabilities and intricacies of the natural world introduce uncertainties. This does not diminish the utility or accuracy of mathematical descriptions of physical laws but highlights the complexities involved in understanding the universe. The effectiveness of mathematics in describing the physical world, despite these uncertainties, remains one of the great mysteries of science.



Einstein himself marveled at this phenomenon, stating in another context, "The most incomprehensible thing about the world is that it is comprehensible." This statement reflects his wonder at the ability of human beings to grasp the workings of the universe through mathematical language, despite the inherent uncertainties when mathematics is applied to the empirical world. This duality suggests that while mathematics provides a remarkably powerful framework for understanding the universe, there remains an element of mystery in how these abstract constructs so accurately capture the behavior of physical systems. It underscores the notion that our mathematical models, as precise as they may be, are still approximations of reality, shaped by human perception and understanding.

Albert Einstein's reflection on the comprehensibility of the world strikes at the heart of a profound philosophical and theological inquiry: if the universe can be understood through the language of mathematics, does this not imply a deliberate design, akin to software engineered to run on the hardware of physical reality? The universe is not a random assembly of laws and constants but a system shaped by a conscious, calculating mind, employing mathematical principles as the foundational 'software' guiding the 'hardware' of the cosmos. The parallel drawn between a software engineer and a divine creator suggests an intentional crafting of the universe, where both the physical laws that govern it and the abstract mathematical principles that describe it are interwoven in a coherent, intelligible framework. This perspective posits that just as an engineer has foresight in designing software and hardware to function in harmony, so too must a higher intelligence have envisioned and instantiated the physical world and its mathematical description. This notion of the universe as a product of design, governed by mathematical laws, implies a creator with an exhaustive understanding of mathematics, one who has encoded the fabric of reality with principles that not only dictate the behavior of the cosmos but also allow for its comprehension by sentient beings. The idea that humans are made 'in the image' of such a creator, with the capacity to ponder the abstract world of mathematics and to 'think God's thoughts after Him,' suggests a deliberate intention to share this profound understanding of the universe. The ability of humans to grasp mathematical concepts, discern the underlying order of the universe, and appreciate the beauty of its design speaks to a shared 'language' or logic between the creator and the created. This shared language enables humans to explore, understand, and interact with the world in a deeply meaningful way, uncovering the layers of complexity and design embedded within the cosmos.

Furthermore, this perspective on the comprehensibility of the world as evidence of a designed universe raises questions about the purpose and nature of this design. It suggests that the universe is not merely a mechanical system operating blindly according to predetermined laws but a creation imbued with meaning, intended to be explored and understood by beings capable of abstract thought and reflection. In this view, the pursuit of science and mathematics becomes not just an intellectual endeavor but a spiritual journey, one that brings humans closer to understanding the mind of the creator. It transforms the study of the natural world from a quest for empirical knowledge to a deeper exploration of the divine blueprint that underlies all of existence. The endeavor to decode the mathematical 'software' of the universe thus becomes an act of communion with the creator, a way to bridge the finite with the infinite and to glimpse the profound wisdom that orchestrated the symphony of creation.




Paul Dirac: God used beautiful mathematics in creating the world

Paul Dirac, a seminal figure in 20th-century physics, is celebrated for his groundbreaking contributions, which have grown increasingly significant over time. His moment of insight, reputedly occurring as he gazed into a fireplace at Cambridge, led to the synthesis of quantum mechanics and special relativity through the formulation of the Dirac equation in 1928. The Dirac equation was a monumental achievement in physics, addressing the need for a quantum mechanical description of particles that was consistent with the principles of relativity. This equation not only accurately predicted the electron's spin but also led to the revolutionary concept of antimatter, fundamentally altering our understanding of the quantum world. Antimatter, as implied by the Dirac equation, consists of particles that mirror their matter counterparts, with the potential for mutual annihilation upon contact, converting their mass into energy in line with Einstein's famous equation, E=mc². This principle also allows for the reverse process, where sufficient energy can give rise to pairs of matter and antimatter particles, challenging the notion of a constant particle count in the universe.

Roger Penrose elaborates on this phenomenon, emphasizing that in such a relativistic framework, the focus shifts from individual particles to quantum fields, with particles emerging as excitations within these fields. This perspective underscores the dynamic and ever-changing nature of the quantum realm, where the creation and annihilation of particles are processes guided by the fundamental laws of physics.  Upon deriving the Dirac equation, which serves as the relativistic framework for describing an electron, we encounter insights into the electron's behavior that illuminate the fundamental properties of matter. A striking revelation emerges when examining the gamma matrices within the equation; their structure aligns with the Pauli spin matrices, responsible for characterizing electron spin. This alignment suggests that the gamma matrices, and thereby the Dirac equation, inherently describe the electron's spin. This discovery was not based on empirical observation but emerged purely from mathematical formalism, showcasing the predictive power of mathematics in elucidating natural phenomena. The Dirac equation's ability to mathematically deduce the concept of electron spin, previously postulated through observational theories, was groundbreaking. This achievement underscored the profound relationship between mathematics and the physical world, revealing the capacity of mathematical theory to predict and explain the workings of nature.

Marco Biagini, a physicist specializing in solid-state physics, posits that the universe's state is governed by specific mathematical laws, suggesting that the universe's existence is contingent upon these equations. Since mathematical equations are abstract constructs originating from a conscious mind, the mathematically structured universe implies the existence of a conscious, intelligent deity conceiving it. This perspective challenges the notion that mathematical equations are mere human representations or languages describing the universe. Instead, it asserts that the intrinsic nature of physical laws as abstract mathematical concepts necessitates an intelligent origin. The precise alignment of natural phenomena with mathematical equations, devoid of any arbitrary "natural principles," points to a universe inherently structured by these equations, further implying a deliberate design by a Creator. The abstract and conceptual nature of the universe's governing laws, as revealed by modern science, is incompatible with atheism, suggesting instead the presence of a personal, intelligent God behind the universe's orderly framework.

Mathematics underlies many natural structures

Fibonacci Sequence and the Golden Ratio: The Fibonacci sequence, where each number is the sum of the two preceding ones (0, 1, 1, 2, 3, 5, 8, 13, ...), appears throughout the natural world. The ratio between successive Fibonacci numbers approximates the Golden Ratio (approximately 1.618), a proportion often found in aesthetically pleasing designs and art. This ratio and sequence are evident in the arrangement of leaves, the branching of trees, the spiral patterns of shells, and even the human body's proportions. The Fibonacci sequence and the Golden Ratio, found in the spirals of shells and the arrangement of leaves, not only contribute to the aesthetic appeal of these forms but also to their functionality. This efficient use of space and resources in plants and animals guided by a mathematical sequence implies a design principle that favors both beauty and utility. Such optimization seems unlikely to arise from random chance, suggesting a deliberate pattern encoded into the very essence of life.

Fractals: Fractals are complex geometric shapes that look the same at every scale factor. This self-similarity is seen in natural structures such as snowflakes, mountain ranges, lightning bolts, and river networks. The Mandelbrot set is a well-known mathematical model that demonstrates fractal properties. Fractals in nature suggest an underlying mathematical rule governing the growth and formation of these structures. Fractals, with their self-similar patterns, demonstrate how complexity can emerge from simple rules repeated at every scale. This phenomenon, manifesting in the branching of trees, the formation of snowflakes, and the ruggedness of mountain ranges, illustrates a principle of efficiency and adaptability. The ability of fractals to model such diverse natural phenomena with mathematical precision points to an underlying order that governs the growth and form of these structures, hinting at a design that accommodates complexity and diversity from simple, foundational rules.



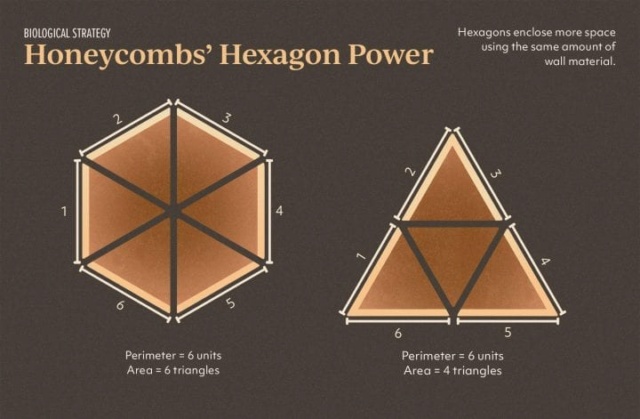
Hexagonal Packing: The hexagon appears frequently in nature due to its efficiency in packing and covering space. The most famous example is the honeycomb structure created by bees, which uses the least amount of material to create a lattice of cells. This geometric efficiency hints at an underlying mathematical principle guiding these natural constructions. Around 36 B.C., Marcus Terentius Varro highlighted the hexagonal architecture of bee honeycombs in his agricultural writings, noting the geometric efficiency of this shape in maximizing space within a circular boundary while preventing contamination from external substances due to the absence of gaps. In a 2019 dialogue, mathematician Thomas Hales, who provided a conclusive proof of this geometric efficiency, emphasized that the hexagonal structure is optimal for covering the largest area with the minimum perimeter. This translates to bees being able to store more honey using less wax for construction, a testament to the efficiency and ingenuity of their natural design. This insight aligns with Charles Darwin's admiration for the honeycomb's design, marveling at its perfect adaptation for its purpose.

David F. Coppedge, in his discussion on honeycombs, contrasts the perceived simplicity of their formation with the precision observed in beehives. While natural phenomena like columnar basalt formations and bubble formations exhibit similar hexagonal patterns due to physical laws, they lack the uniformity and purposefulness evident in honeycombs, which are meticulously constructed for specific functions like honey storage and brood rearing. The distinction between natural formations and those crafted with intent is further illustrated through the comparison of natural and human-made arches. Natural arches, formed by erosion, lack a defined purpose, whereas human-engineered arches like the Arc de Triomphe or Roman aqueducts serve specific functions and are built with precise specifications, showcasing the role of intelligent design. The honeycomb's precise geometry, far from being a mere byproduct of physical laws, suggests a deliberate engagement with natural principles. Bees, by leveraging surface tension in their construction process, demonstrate not just an instinctual behavior but a sophisticated interaction with the natural world that reflects purpose and design. This interplay between natural law and biological instinct underscores the complexity and wonder of natural structures, inviting deeper exploration into the origins and mechanisms of such phenomena. The hexagonal packing in honeycombs exemplifies geometric efficiency, where bees construct their hives using the least amount of wax to create the maximum storage space. This not only showcases an understanding of spatial optimization but also suggests a principle of economy and sustainability in nature's design. The meticulous precision of these structures contrasts sharply with the irregular forms produced by similar physical processes without biological intervention, like the formation of columnar basalt. This discrepancy raises questions about the source of nature's apparent ingenuity and foresight, which seem to eclipse the capabilities of blind physical forces.

Phyllotaxis: This term refers to the arrangement of leaves on a stem or seeds in a fruit, which often follows a spiral pattern that can be modeled mathematically. The angles at which leaves are arranged maximize sunlight exposure and minimize shadow cast on other leaves, suggesting an optimized design governed by mathematical rules. Phyllotaxis and the arrangement of leaves or seeds follow mathematical patterns that optimize light exposure and space usage, demonstrating a sophisticated understanding of environmental conditions and resource management. This level of optimization for survival and efficiency suggests a preordained system designed with the well-being and prosperity of organisms in mind.

Wave Patterns: The mathematics of wave patterns can be observed in various natural phenomena, from the ripples on a pond's surface to the sand dunes shaped by wind. The study of these patterns falls under the field of mathematical physics, where equations such as the Navier-Stokes equations for fluid dynamics describe the movement and formation of waves.

Crystal Structures: The atomic arrangements in crystals often follow precise mathematical patterns, with regular geometrical shapes like cubes, hexagons, and tetrahedrons. These structures are determined by the principles of minimum energy and maximum efficiency, hinting at an underlying mathematical order.

Voronoi Diagrams: These are mathematical partitions of a plane into regions based on distance to points in a specific subset of the plane. Natural examples of Voronoi patterns can be seen in the skin of giraffes, the structure of dragonfly wings, and the cellular structure of plants. Voronoi diagrams illustrate how nature efficiently partitions space, whether in the territorial patterns of animals or the microscopic structure of tissues. This spatial organization, governed by mathematical rules, ensures optimal resource allocation and interaction among system components, further emphasizing a principle of intentional design for functionality and coexistence.

The patterns and structures observed in nature, which are so elegantly described by mathematical principles, invite reflection on the origins and underlying order of the universe. The prevalence of mathematical concepts like the Fibonacci sequence, fractals, hexagonal packing, and others in the natural world suggests more than mere coincidence; it hints at an intentional design woven into the fabric of reality. Moreover, the mathematical description of wave patterns and the structured atomic arrangements in crystals reveal a universe where the fundamental laws governing the cosmos are rooted in mathematical concepts. These laws facilitate the formation of stable, ordered structures from the microscopic to the cosmic scale, embodying principles of harmony and balance that seem too deliberate to be the product of random events. When viewed through the lens of these mathematical principles, the natural world appears not as a collection of random, isolated phenomena but as a coherent, interconnected system shaped by a set of fundamental rules that hint at purposeful design. The pervasive use of mathematics in describing natural phenomena suggests an architect behind the cosmos, one who employs mathematical laws as the blueprint for the universe. This perspective invites a deeper exploration of the origins and meaning of the natural order, pointing towards a designed universe that transcends the capabilities of chance and necessitates a guiding intelligence.

Objection: Mathematics and physics describe the natural phenomena of the universe; the universe would exist whether or not we could describe it with any degree of accuracy.
Answer: The mathematical rules that underpin the physical world are instructional software, guiding the behavior and interaction of matter and energy across the universe. This perspective highlights the profound role of mathematics as the language of nature, providing a structured framework that dictates the fundamental laws and constants governing everything from the subatomic scale to the cosmic expanse. At the heart lies the concept that just as software contains specific instructions to perform tasks and solve problems within a predefined framework, the mathematical principles inherent in the universe serve as the instructions for how physical entities interact and exist. These principles are not just abstract concepts but are deeply embedded in the fabric of reality, dictating the structure of atoms, the formation of stars, the dynamics of ecosystems, and the curvature of spacetime itself. For instance, consider the elegant equations of Maxwell's electromagnetism, which describe how electric and magnetic fields propagate and interact. These equations are akin to a set of programming functions that dictate the behavior of electromagnetic waves, influencing everything from the transmission of light across the cosmos to the electrical impulses in our brains. Similarly, the laws of thermodynamics, which govern the flow of energy and the progression of order to disorder, can be likened to fundamental operating principles embedded in the software of the universe. These laws ensure the directionality of time and the inevitable march towards equilibrium, influencing the life cycle of stars, the formation of complex molecules, and the metabolic processes fueling life. On a grander scale, Einstein's equations of General Relativity provide the 'code' that describes how mass and energy warp the fabric of spacetime, guiding the motion of planets, the bending of light around massive objects, and the expansion of the universe itself. These equations are like deep algorithms that shape the very geometry of our reality, influencing the cosmic dance of galaxies and the intense gravity of black holes.

Furthermore, in the quantum realm, the probabilistic nature of quantum mechanics introduces a set of rules that are the subroutines governing the behavior of particles at the smallest scales. These rules dictate the probabilities of finding particles in certain states, the strange entanglement of particles over distances, and the transitions of atoms between energy levels, laying the foundation for chemistry, solid-state physics, and much of modern technology.
This 'instructional software' of the universe, written in the language of mathematics, underscores a remarkable order and predictability amidst the vast complexity of the cosmos. It reveals a universe not as a chaotic ensemble of particles but as a finely tuned system governed by precise laws, enabling the emergence of complex structures, life, and consciousness. The pursuit of understanding these mathematical 'instructions' drives much of scientific inquiry, seeking not only to decipher the code but to comprehend the mind of the universe itself. The mathematical framework that serves as the 'instructional software' for the universe, guiding everything from the movement of subatomic particles to galaxies, is evidence of a universe governed by an intelligible set of principles. However, there's no underlying necessity dictating that the universe must adhere to these specific rules. The mathematical laws we observe, from quantum mechanics to general relativity, could be different, or could not exist at all, leading to a universe vastly different from our own, potentially even one where life as we know it could not emerge. This recognition opens a philosophical and scientific inquiry into the "why" behind the universe's particular set of rules. The constants and equations that form the bedrock of physical reality, such as the gravitational constant or the fine structure constant, are finely tuned for the emergence of complex structures, stars, planets, and ultimately life. If these fundamental constants were even slightly different, the delicate balance required for the formation of atoms, molecules, and larger cosmic structures could be disrupted, rendering the universe sterile and void of life. The absence of a deeper, underlying principle that mandates the universe's adherence to these specific mathematical rules invites to a reflection. It raises questions about the nature of reality and our place within it. Why does the universe follow these rules?  The realization that the universe could have been different, yet operates according to principles that allow for the complexity and richness of life, points to the instantiaton of these laws by a law-giver, we commonly call God.



Decoding reality - Information is fundamental

Since ancient Greek times, Western thinkers have predominantly held two major perspectives and worldviews on the fundamental nature of existence. One dominant perspective posits that consciousness or mind is the foundational reality. From this viewpoint, the physical universe either emanates from a pre-existing consciousness or is molded by a prior intelligence or both. Consequently, it is the realm of the mind, rather than the physical, that is deemed the primary or ultimate reality—the origin or the force capable of influencing the material universe. Philosophers such as Plato, Aristotle, the Roman Stoics, Jewish thinkers like Moses Maimonides, and Christian scholars such as St. Thomas Aquinas have each embraced variants of this notion. This mindset was also prevalent among many pioneers of modern science during the era known as the scientific revolution (1300–1700), who believed their exploration of nature validated the existence of an “intelligent and powerful Being” as articulated by Sir Isaac Newton, underlying the universe. This philosophical stance is often termed idealism, highlighting the primacy of ideas over physical matter.

In 1610, Rene Descartes, a famous French philosopher, and mathematician, embarked on a quest to establish a foundational argument for the existence of the human soul, and subsequently, the existence of God and His dominion over the material world. Descartes realized that while the authenticity of his sensory experiences could be questioned, the very act of doubting was undeniable. His famous conclusion, "Cogito, ergo sum" or "I think, therefore I am," served as a pivotal religious assertion, affirming the existence of the human spirit from which he derived the presence of God. Descartes posited a dualistic nature of reality, where spiritual entities were distinct from material ones, the latter being inert and devoid of intellect or creativity, qualities he attributed solely to the divine. This perspective, however, was overshadowed during the Enlightenment, a period that ironically neglected Descartes' primary message. Instead, the era emphasized human reason as the cornerstone of knowledge and portrayed the universe as a vast mechanical system governed by immutable laws, negating the possibility of divine interventions.

Isaac Newton, another devout Christian and a luminary in physics perceived the universe as a meticulously orchestrated mechanism functioning under divine laws. Yet, Newton recognized the existence of "active" principles, such as gravity and magnetism, which he interpreted as manifestations of divine influence on the physical realm. To Newton, gravitational forces exemplified God's meticulous governance of the cosmos, with the orderly nature of the universe and the solar system serving as a testament to intelligent design. However, much like Descartes, Newton's intentions were gradually overlooked, leading to a materialistic interpretation of his theories, contrary to his original apologetic stance against such a worldview. This misconstrued "Newtonian" perspective, erroneously associated with Newton himself, prioritized the physical dimensions and dismissed the realms of mind and spirit, a narrative popularized not by scientists but by literary figures and philosophers like Fontanelle and Voltaire. The Enlightenment era, thus, ushered in a philosophy that championed human "Reason" as the bedrock of all understanding, reducing human thoughts and sensations to mere mechanical interactions of brain atoms. J.O. de La Mettrie's bold proclamation that "man is a machine" and his dismissal of the practical relevance of a supreme being's existence marked a significant pivot towards naturalism, underscoring the profound transformation of the original ideas posited by Descartes and Newton in the Enlightenment's intellectual landscape. While some individuals find the naturalistic worldview satisfying, it is in reality fraught with contradictions and inconsistencies, failing to align with various scientific observations and human experiences, and challenging to consistently apply in practice. This perspective, upon examination, appears to falter under critical truth assessments. A fundamental tenet of naturalism posits that only matter and energy exist, either created spontaneously from nothing, or existing eternally, implying that human consciousness and thought are merely byproducts of material processes. This raises a critical question: if human thoughts are solely the outcome of material interactions within the brain, how can we trust these thoughts to accurately reflect reality? The inherent nature of matter does not include a predisposition towards truth, casting doubt on the reliability of perceptions and beliefs derived from purely material processes. 

Convergence of Consciousness with the Quantum World

The composition of matter can be traced from the macroscopic level down to the microscopic realm through a hierarchical structure: Matter consists of molecules, which are in turn composed of atoms. Atoms are made up of electrons orbiting nuclei, which themselves consist of protons and neutrons. This reductionist view, tracing back to ancient Greek philosophy, posits that there is a fundamental level of indivisible particles that serve as the ultimate building blocks of all matter. The quest for these elementary particles has led to the development of quark theory, which is currently supported by substantial experimental evidence. Quark theory introduces quarks as the fundamental constituents of protons and neutrons, suggesting that these subatomic particles are not indivisible but are composed of even smaller entities. Quarks are conceived as point-like particles that possess unique properties, including charge, mass, and spin, as well as a characteristic known as "color" which is central to the theory of quantum chromodynamics (QCD). QCD is the field of physics that describes the strong force, one of the four fundamental forces of nature, which is responsible for binding quarks together within protons, neutrons, and other hadrons. Quark theory not only provides a more fundamental understanding of matter's structure but also opens up questions about the nature of these elementary particles and the forces that govern their interactions. It represents a significant milestone in the ongoing effort to understand the universe's most basic components, pushing the boundaries of how we conceptualize the fabric of reality. 

Some theorists propose an alternative to the concept of a divine first cause by suggesting that the universe originated from energy existing eternally within a singularity, with a quantum fluctuation triggering the cosmos's sudden emergence. This perspective contrasts with Planck's view, which posited that consciousness is the basis from which matter and energy derive. The inverse proposition, where consciousness emerges from energy, implies that over an undefined span of time, energy could develop cognitive and self-aware characteristics, potentially acquiring the capacity for perception, thought, volition, ethical discernment, and memory. However, this hypothesis encounters significant philosophical hurdles, as it presupposes the pre-existence of logical frameworks necessary for cognitive processes such as differentiation, syllogistic reasoning, and both inductive and deductive logic. Einstein highlighted a critical flaw in this materialistic viewpoint, pointing out the profound "gulf" that exists between the tangible realm of physical objects and the intangible domain of ideas and abstractions. One of the challenges for materialism is explaining how inanimate matter can spontaneously give rise to abstract concepts and consciousness, a transformation that has not been observed or demonstrated empirically. This gap underscores the complexities and mysteries surrounding the origins and nature of consciousness and its relationship with the physical universe.

Max Planck, theoretical physicist who originated quantum theory, which won him the Nobel Prize in Physics in 1918
“I regard consciousness as fundamental. I regard matter as derivative from consciousness. We cannot get behind consciousness. Everything that we talk about, everything that we regard as existing, postulates consciousness.” 

Eugene Wigner, theoretical physicist and mathematician. He received a share of the Nobel Prize in Physics in 1963
“It was not possible to formulate the laws of quantum mechanics in a fully consistent way without reference to consciousness.”

R.C. Henry,  Professor of Physics and Astronomy at Johns Hopkins University ,  “The Mental Universe” ; Nature 436:29,2005) ?  He wrote:
“A fundamental conclusion of the new physics also acknowledges that the observer creates the reality. As observers, we are personally involved with the creation of our own reality. Physicists are being forced to admit that the universe is a “mental” construction.

Sir Arthur Eddington explained:
“It is difficult for the matter-of-fact physicist to accept the view that the substratum of everything is of mental character.”

R.C. Henry, Professor of Physics and Astronomy at Johns Hopkins University , “The Mental Universe” ; Nature 436:29,2005) Link
“A fundamental conclusion of the new physics also acknowledges that the observer creates the reality. As observers, we are personally involved with the creation of our own reality. Physicists are being forced to admit that the universe is a “mental” construction. Pioneering physicist Sir James Jeans wrote: “The stream of knowledge is heading toward a non-mechanical reality; the universe begins to look more like a great thought than like a great machine. Mind no longer appears to be an accidental intruder into the realm of matter, we ought rather hail it as the creator and governor of the realm of matter. Get over it, and accept the inarguable conclusion. The universe is immaterial-mental and spiritual.” 

Paul Davies, the director of BEYOND, highlights a paradigm shift occurring within the physics community, where a growing number of physicists are reconsidering the foundational elements of the universe. Traditionally, matter has been viewed as the primary substance from which everything else—forces, energy, even space-time—emerges. Information, on the other hand, was seen more as a byproduct or a secondary attribute of the physical world. However, this traditional view is being challenged by the notion that at the most fundamental level, the universe might be fundamentally composed of information and the processes by which this information is organized, manipulated, and transformed. In this emerging perspective, matter itself is seen as a manifestation or emergent property of underlying informational processes. This suggests that what we perceive as physical might not be as solid or fundamental as we have believed. Expanding on this, if we entertain the idea that information is the bedrock of reality, it opens up philosophical and metaphysical inquiries that go beyond conventional scientific discourse. One intriguing proposition is that matter, energy, and even space might not possess an intrinsic physical structure but could be manifestations of something more abstract, akin to the way images on a computer screen emerge from binary code. This line of thinking aligns with some interpretations of quantum mechanics, where particles do not have definite states until they are observed, suggesting a reality that is dependent on information rather than independent physical entities. In such a framework, the physical universe might be akin to a holographic projection, where depth, solidity, and separation are experienced perceptually but might not reflect an underlying physical truth.

Taking this a step further, if we consider information as the fundamental substrate of reality, it leads to speculative yet fascinating questions about the nature of consciousness and the origins of this information. It posits that perhaps our universe, with all its complexity and intricacy, could be the product of a conscious mind—an ultimate intelligence or a "Prime Creator." In this view, what we perceive as physical reality could be akin to thoughts or ideas within the mind of this Prime Creator, where matter, energy, and space are not tangible entities but the expressions of a divine consciousness. This perspective resonates with some ancient philosophical and spiritual traditions that view the material world as an illusion or Maya, suggesting that underlying the world of phenomena is a deeper, more fundamental reality that is beyond the physical. In such a view, science and spirituality converge on the notion that the essence of all existence might be informational or consciousness-based, rather than material. This radical reimagining of the universe's foundation challenges our most basic assumptions about reality and invites a deeper exploration of the nature of existence itself. The theistic view, in contrast to the naturalistic one, posits that information, rather than matter or energy is the foundational element from which everything in the universe is constructed. This perspective holds that the origins and behaviors of phenomena at the microscopic level, like energy and matter, can be elucidated through information. However, as highlighted by thinkers such as Deutsch and Wheeler, any proposed fundamental element of the universe must also account for its own genesis. This brings us to the puzzle of how something can emerge from nothing, or how initial information can spring from a state of absolute void. This leads to an overarching question: Is it possible that a singular, all-encompassing principle or a "master equation" exists that can describe the entirety of the universe? At the heart of this exploration is the most captivating and fundamental inquiry: Why does reality exist, and what is its source? Before going into the interconnectedness of things, we must confront the more primal question of their very existence. Framing reality in terms of 'information bits' not only offers insights into why reality exists but also clarifies how everything within it is interconnected, making the nature of existence and its network of relationships evident.

In physics and computer science, "information" is a well-defined concept with specific properties and behaviors. Extending the concept of information to be the foundational element of physical reality requires redefining what information means in a way that encompasses physical laws and phenomena. Skeptics argue that this redefinition is not straightforward and could stretch the concept of information beyond its useful or meaningful application. The prevailing view in science holds that everything that exists is physical in nature. This view is deeply ingrained in the scientific method and is supported by a vast body of research and experimentation. Critics argue that redefining fundamental reality as non-physical (information or consciousness) without concrete physical evidence challenges the very basis of scientific inquiry. Idealism, the philosophical stance that posits consciousness or mind as the foundational substance of reality, faces significant hurdles when reconciled with contemporary scientific understanding and empirical methodology. While idealism offers a radical reimagining of the nature of existence, positioning consciousness at the heart of everything, this perspective diverges markedly from the frameworks that underpin much of modern science.



The core question in this exploration is whether a singular, overarching principle exists that can encapsulate the entirety of the universe. Central to this inquiry is the fundamental question: Why does reality exist, and from where did it originate? To address the interconnectedness within the universe, we must first grapple with the more fundamental query of existence itself. The concept of 'information' offers insights into both these questions. Information is posited as a more elementary aspect of the universe than either matter or energy. Viewing the universe through the lens of 'information bits' sheds light on both the existence of reality and the web of connections within it, making these concepts appear much more intuitive. In a philosophical stance, which veers away from traditional materialism, the physical matter outlined by the Standard Model of Physics is a secondary phenomenon that emerges from an underlying informational framework. The notion that our universe might resemble a hologram is increasingly gaining traction. With advancements in quantum computing, physicists are poised to substantiate this theory with compelling evidence.

In 2015, an interesting perspective was offered, challenging conventional notions about the fundamental components of the cosmos. Instead of atoms, particles, energy, quantum mechanics, forces, fields, or the fabric of space-time, "information" was proposed as the core element of reality. Echoing the late esteemed physicist John Archibald Wheeler's "It from bit" principle, this view posits that all entities in the universe, or 'it', are essentially derived from informational units, or 'bits'. Paul Davies articulates a shift in scientific perspective, where traditionally, matter was seen as the primary substance, with information being a derivative. In contrast, a growing faction of physicists now suggests inverting this hierarchy. They propose that at the most fundamental level, the universe might be fundamentally about information and its processing, with matter emerging as a consequent notion.

Seth Lloyd, a quantum information specialist from MIT, proposes an intriguing analogy, suggesting that the universe operates much like a computer. He explains this by pointing out that electrons exhibit spin, which quantum mechanics tells us can be in one of two states: either 'up' or 'down'. These two states bear a striking resemblance to the binary system used in computing, with bits representing the two states. Lloyd posits that at its most fundamental level, the universe is made up of information, with each elementary particle serving as a carrier of information. He poses the question, "What is the universe?" and answers it by describing the universe as a physical system that systematically organizes and processes information, capable of performing any computation a computer can. Lloyd takes this analogy further, suggesting that the universe's operation as a computer is not just a metaphorical framework but a literal description of how the universe functions. In his view, every transformation within the universe can be seen as a form of computation, making this perspective a significant claim in the field of physics. Echoing this sentiment, physicist Stephen Wolfram, known for creating Mathematica and Wolfram Alpha, highlights information as a fundamental concept in our era. He suggests that the complexity observed in nature can be traced back to simple rules, which he believes are best understood through the lens of computation. According to Wolfram, these simple computational rules fundamentally underpin the universe.



The Biocentric Universe Theory offers a different take, arguing that life itself gives rise to the constructs of time, space, and the cosmos. This idea challenges the notion of time as an independent entity, instead suggesting that our perception of time is intrinsically linked to life's observational capacities. Illustrating this proposition, consider watching a film of an archery tournament. If the film is paused, the arrow in mid-flight appears frozen, allowing precise determination of its position but at the loss of information about its momentum. This scenario draws parallels to Heisenberg’s uncertainty principle, which posits that measuring a particle's position inherently compromises knowledge of its momentum, and vice versa. From a biocentric viewpoint, our perceptions, including time and space, are not external realities but are continually reconstructed within our minds from information. Time is perceived as a series of spatial states processed by the mind. Therefore, what we perceive as reality is a function of changing mental images. This perspective argues that what we attribute to an external 'time' is merely our way of interpreting changes. Similarly, space is not considered a physical entity but a framework within which we organize our sensory experiences, further emphasizing the central role of information in shaping our understanding of the universe.

Many of us still adhere to a Newtonian concept of space, imagining it as a vast, wall-less container. However, this traditional view of space is fundamentally flawed. Firstly, the concept of fixed distances between objects is undermined by Einstein's theory of relativity, which shows that distances can change based on factors like gravity and velocity, eliminating the idea of absolute distance. Secondly, what we perceive as empty space is, according to quantum mechanics, teeming with potential particles and fields, challenging the notion of emptiness. Thirdly, the principle of quantum entanglement suggests that particles can remain connected and influence each other instantaneously over vast distances, questioning the idea of separation. In his work "INFORMATION–CONSCIOUSNESS–REALITY," James B. Glattfelder introduces the provocative notion that consciousness might be a fundamental aspect of the universe. Historically, physics has considered elements like space, time, and mass as fundamental, with laws such as gravity and quantum mechanics governing them without being reducible to simpler principles. Glattfelder argues that consciousness, much like electromagnetic phenomena in Maxwell's era, cannot be explained by existing fundamentals and thus should be considered a fundamental entity. This perspective doesn't exclude consciousness from scientific inquiry; rather, it provides a new foundational element for exploration. Glattfelder further suggests that the connection between consciousness and physical processes might be best understood through the lens of information processing. This implies a spectrum of consciousness tied to the complexity of information processing, ranging from the simple to the highly complex. This view aligns with observations from physicists and philosophers who note the abstract nature of physics, which describes the structure of reality through equations without addressing the underlying essence. Stephen Hawking's query about what "puts the fire into the equations" points to a deeper inquiry about the essence of reality. According to this perspective, it is consciousness that animates the equations of physics, suggesting that the flux of consciousness is what physics ultimately describes, offering a profound connection between consciousness, information, and the fabric of reality.



In the realm of quantum physics, the nature of matter is profoundly redefined, challenging our classical perceptions. Renowned scientists have delved into the atomic and subatomic levels, revealing that what we consider matter does not exist in a traditional, tangible sense. Instead, matter is the manifestation of underlying forces that cause atomic particles to vibrate and bind together, forming what we perceive as the physical world. This leads to the postulation that a conscious, intelligent force underpins these fundamental interactions, serving as the fabric from which all matter is woven. Werner Heisenberg, a central figure in quantum mechanics, observed that atoms and elementary particles are not concrete entities but represent a realm of possibilities or potentialities, challenging the notion of a fixed, material reality. Reflecting on these insights, it becomes evident that the smallest constituents of matter are better described as mathematical forms or ideas, rather than physical objects in the conventional sense. This perspective aligns with Platonic philosophy, where abstract forms or ideas are the ultimate reality.

Sir James Hopwood Jeans
Today there is a wide measure of agreement, which on the physical side of science approaches almost to unanimity, that the stream of knowledge is heading towards a non-mechanical reality; the universe begins to look more like a great thought than like a great machine. Mind no longer appears as an accidental intruder into the realm of matter; we are beginning to suspect that we ought rather to hail it as a creator and governor of the realm of matter... 5

Quantum physics further unveils that atoms are composed of dynamic energy vortices, continuously in motion and emanating distinct energy signatures. This concept, often referred to as "the Vacuum" or "The Zero-Point Field," represents a sea of energy that underlies and sustains the physical universe, highlighting the ephemeral and interconnected nature of what we call matter.

Regarding energy, traditionally defined in physics as the capacity to do work or induce heat, the question arises why it is described merely as a "property" rather than a more active, dynamic force. This inquiry opens the door to more metaphysical interpretations, such as considering energy as the active expression of fundamental universal principles, akin to the "word" in theological contexts, where the spoken word carries the power of creation and transformation. In this light, matter, often perceived as static and tangible, is reinterpreted as a manifestation of energy, emphasizing the fluid and interconnected nature of all that exists. This perspective invites a broader, more holistic view of the cosmos, where the distinctions between matter, energy, and information are seen as different expressions of a unified underlying reality.

Hebrews 11:3: By faith we understand that the universe was formed at God’s command so that what is seen was not made out of what was visible.
Acts 17:28: For in Him we live and move and have our being, as also some of your own poets have said, ‘For we are also His offspring.’
Romans 11:36 For from him and through him and for him are all things.
John 1:3 Through him all things were made; without him nothing was made that has been made.
Colossians 1:16 For in him all things were created: things in heaven and on earth, visible and invisible, whether thrones or powers or rulers or authorities; all things have been created through him and for him.



The argument of the Mind Over Matter

Newton contended that atheism often stems from the belief that physical entities possess an inherent, complete reality independent of any external influence.
The advent of quantum mechanics in 1925 revolutionized our understanding of the universe's nature, nudging some of the brightest physicists towards a paradigm that might seem implausible to atheists: the notion that the universe is fundamentally mental in nature.
Sir James Jeans, a distinguished figure in astronomy, mathematics, and physics at Princeton University, observed a shift in scientific understanding towards a non-mechanical reality. He suggested that the universe more closely resembles a grand thought rather than a vast machine, proposing that consciousness is not merely a random occurrence within matter but rather its creator and orchestrator.
The argument posits that inanimate matter alone cannot give rise to consciousness. For instance, even if all the components of the brain were assembled under natural conditions, consciousness or a mind would not spontaneously emerge from mere physical interactions. In contrast, a conscious mind is capable of creating organized structures, such as a computer, from inanimate matter.
From this perspective, it follows that consciousness or mind must have existed prior to material reality.
It is proposed that the mind is an attribute of a conscious being, and thus, the universal consciousness could be attributed to a divine entity.
The conclusion drawn from this line of reasoning is the affirmation of God's existence.