Pauli's Exclusion principle: The biggest deal of all in quantum physics
https://reasonandscience.catsboard.com/t3148-pauli-s-exclusion-principle-the-biggest-deal-of-all-in-quantum-physics
The most common fermion to our everyday life is the electron, and this packing limitation of electrons has a profound influence on our world, effectively defining the subject of chemistry. This is the famous Pauli exclusion principle in action. Electrons live in certain energy levels about atoms, with two in the lowest level of an atom, known as the ground state. Why two? Electrons possess a quantized spin of ½,
The fundamental tenet of quantum mechanics is that many basic phenomena at the atomic level (such as energy emission and angular momentum) appear to be quantized. “Quantized” means that when certain properties of a system are measured, a continuous range of results are not observed, but rather a set of discrete (separate) values. If “quanta” come in discrete units then it makes sense to count them with integers.
The idea of a spin = ½ particle was at first met with skepticism by much of the scientific community. It was not until 1928 when Paul Dirac directly derived this result for the electron from relativistic quantum mechanics that half-integer quantum numbers became widely accepted.
http://mriquestions.com/why-i--frac12-1-etc.html
which can point either upwards or downwards, and so the lowest energy state can be occupied by one electron with its spin pointing upwards, as well as a second electron with its spin pointing downwards. (Classically, we define the direction of a spin with the right-hand-rule: wrap your right hand around the spinning object, with your fingers pointing with the spin. Give a thumbs-up; this defines the direction of the spin). In terms of packing electrons, this lowest level of the atom is full, and any additional electrons must go into higher levels. And as these upper levels fill, incoming electrons settle into higher and higher energy levels. What does this have to do with chemistry? Well, chemistry is about how atoms interact, and this is completely defined by the locations of the electrons in the outermost energy levels; these are the most loosely bound electrons, and it is these that can be swapped between atoms to allow them to bond and form complex molecules. It is these electrons, and the properties of their spin and orbits, that give atoms their personality. The same is true of atomic nuclei. Protons and neutrons, themselves fermions, can only be found on distinct energy levels with the nucleus, packed in accordance with the Pauli exclusion principle. This explains why, while isolated neutrons rapidly decay, a neutron inside an atomic nucleus is effectively stable. It cannot decay because no lower energy positions are available for the resultant proton. What if electrons were bosons rather than fermions? The result would be disastrous as there would be nothing to prevent all the electrons occupying the lowest level in an atom, like stuffing as many photons in the box as you can. Once again, wave chemistry – and the chemical complexity and flexibility needed by life – goodbye. These bosonic electrons would be very tightly bound to their nuclei, with little inclination to be shared with other atoms. This universe would be a sea of individual atoms floating through the cosmos, minding their own business and not getting involved in this messy work of forming molecules. However, the situation could be even more complicated! As well as the electrons, the quarks are also fermions, also possessing a spin of one-half. And this spin is imprinted (in a rather complex fashion) onto the particle they form. And both the proton and the neutron, even though they are composite objects, have a total spin of one-half, also making them fermions, and ensuring they obey the fermion rules of packing. This means that the protons and neutrons of an atom are arranged in orbits very similar to the orbits of the much more distant electrons. If quarks, and so also the protons and neutrons, had an integer spin, just like the electrons, there would be nothing to prevent all of them collapsing down and occupying the lowest energy level. Collapsing all of the protons and neutrons in your atoms is not necessarily a catastrophe; there are more important things to worry about.
If Pauli exclusion principle did not exist, we would not have a stable atom and hence no chemistry. Ethan Siegel calls this the biggest deal of all.
CALUM MILLER Defence of the fine-tuning argument JULY 25, 2017
Bohr’s Quantization Rule
Electrons can be seen as planet-like entities that orbit the nucleus but only at explicit energy levels that are governed by straightforward mathematical rules.
Danish physicist Niels Bohr proposed this at the beginning of the 20th century, suggesting that electrons can only occupy discrete orbitals around atoms. If this were not the case, then electrons would gradually reduce their energy (by radiation) and eventually (though very rapidly) lose their orbits. This would preclude atomic stability and chemical complexity, and so also preclude the existence of EMAs.
The Pauli Exclusion Principle
Newscientist (2014): Without the Pauli exclusion principle, matter as we know it would not exist. Named after the Austrian physicist Wolfgang Pauli, who proposed it in 1925, it says that no two electrons in an atom can enter the same quantum state. This leads to atomic electrons filling an elaborate structure of higher and higher energies around the nucleus, explaining why atoms with different numbers of electrons have different properties. Without the Pauli exclusion principle, chemical elements wouldn’t exist. The principle applies not just to electrons, but to all the particles that make up conventional matter, collectively known as fermions. Its influence extends even to the stars.
E.Siegel If it weren't for the Pauli Exclusion Principle, the matter we have in our Universe would behave in an extraordinarily different fashion. The electrons, you see, are examples of fermions. Every electron is fundamentally identical to every other electron in the Universe, with the same charge, mass, lepton number, lepton family number, and intrinsic angular momentum (or spin).
Princeton physicist Freeman Dyson has pointed out (1979, p. 251), if the Pauli exclusion principle did not exist—which is what keeps two electrons from occupying the same energy state in an atom—all electrons would occupy the lowest atomic energy state, and thus no complex atoms could exist. Thus, if any of these fundamental laws or principles were missing, the existence of complex, intelligent life would probably be rendered impossible.
This principle, formalized in 1925 by Austrian physicist Wolfgang Pauli, says that no two particles with half-integer spin (fermions) can occupy the same quantum state at the same time. Since each orbital has only two possible quantum states, this implies that only two electrons can occupy each orbital. This prevents electrons from all occupying the lowest atomic orbital, and so facilitates complex chemistry
https://calumsblog.com/2017/07/25/full-defence-of-the-fine-tuning-argument-part-4/
Finally, consider the Pauli Exclusion Principle, which dictates that no two fermions (spin-½ particles) can occupy the same quantum state. This arises from a deep principle in quantum mechanics which requires that the joint wave function of a system of fermions be antisymmetric. This implies that not more than two electrons can occupy the same orbital in an atom, since a single orbital consists of two possible quantum states (or more precisely, eigenstates) corresponding to the spin pointing in one direction and the spin pointing in the opposite direction. This allows for complex chemistry since without this principle, all electrons would occupy the lowest atomic orbital. Thus, without this principle, no complex life would be possible.
https://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.696.63&rep=rep1&type=pdf
Luke A. Barnes The Fine-Tuning of the Universe for Intelligent Life June 11, 2012
If electrons were bosons, rather than fermions, then they would not obey the Pauli exclusion principle. There would be no chemistry.
https://arxiv.org/pdf/1112.4647.pdf
Stephen C. Meyer: The return of the God hypothesis, page 189
The operation of a principle in the physical world such as the Pauli exclusion principle that (a) enables complex material structures to form and yet (b) limits the atomic weight of elements (by limiting the number of neutrons in the lowest nuclear shell). Thus, the forces at work in the universe itself (and the mathematical laws of physics describing them) display a fine-tuning that requires explanation. Yet, clearly, no physical explanation of this structure is possible, because it is precisely physics (and its most fundamental laws) that manifests this structure and requires explanation. Indeed, clearly physics does not explain itself. See Gordon, “Divine Action and the World of Science,” esp. 258–59; Collins, “The Fine-Tuning Evidence Is Convincing,” esp. 36–38.
If electrons were bosons, rather than fermions, then they would not obey the Pauli exclusion principle. There would be no chemistry. It implies that not more than two electrons can occupy the same orbital in an atom, since a single orbital consists of two possible quantum states corresponding to the spin pointing in one direction and the spin pointing in the opposite direction. This allows for complex chemistry since, without this principle, all electrons would occupy the lowest atomic orbital. Thus, without this principle, no complex life would be possible.
R. P. Feynman wrote of the Pauli exclusion principle, “In fact, almost all the peculiarities of the material world hinge on this wonderful fact.” Measurements show no violation and confirm the generalized Pauli exclusion principle with an error of one part in one quintillion.
https://www.nature.com/articles/s42005-019-0110-3
S. Hossenfelder (2020): The Pauli exclusion principle is a law of nature; it's just how the world is.
https://www.frontiersin.org/articles/10.3389/fphy.2020.00139/full
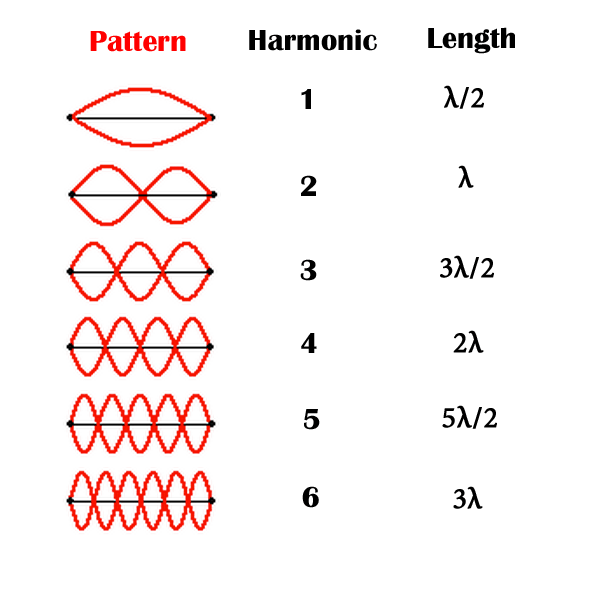
Electrons in an atom form what are known as standing waves, which just means a wave confined in a certain area. Electrons in atoms form three-dimensional standing waves called spherical harmonics.
https://reasonandscience.catsboard.com/t3148-pauli-s-exclusion-principle-the-biggest-deal-of-all-in-quantum-physics
The most common fermion to our everyday life is the electron, and this packing limitation of electrons has a profound influence on our world, effectively defining the subject of chemistry. This is the famous Pauli exclusion principle in action. Electrons live in certain energy levels about atoms, with two in the lowest level of an atom, known as the ground state. Why two? Electrons possess a quantized spin of ½,

The fundamental tenet of quantum mechanics is that many basic phenomena at the atomic level (such as energy emission and angular momentum) appear to be quantized. “Quantized” means that when certain properties of a system are measured, a continuous range of results are not observed, but rather a set of discrete (separate) values. If “quanta” come in discrete units then it makes sense to count them with integers.
The idea of a spin = ½ particle was at first met with skepticism by much of the scientific community. It was not until 1928 when Paul Dirac directly derived this result for the electron from relativistic quantum mechanics that half-integer quantum numbers became widely accepted.
http://mriquestions.com/why-i--frac12-1-etc.html
which can point either upwards or downwards, and so the lowest energy state can be occupied by one electron with its spin pointing upwards, as well as a second electron with its spin pointing downwards. (Classically, we define the direction of a spin with the right-hand-rule: wrap your right hand around the spinning object, with your fingers pointing with the spin. Give a thumbs-up; this defines the direction of the spin). In terms of packing electrons, this lowest level of the atom is full, and any additional electrons must go into higher levels. And as these upper levels fill, incoming electrons settle into higher and higher energy levels. What does this have to do with chemistry? Well, chemistry is about how atoms interact, and this is completely defined by the locations of the electrons in the outermost energy levels; these are the most loosely bound electrons, and it is these that can be swapped between atoms to allow them to bond and form complex molecules. It is these electrons, and the properties of their spin and orbits, that give atoms their personality. The same is true of atomic nuclei. Protons and neutrons, themselves fermions, can only be found on distinct energy levels with the nucleus, packed in accordance with the Pauli exclusion principle. This explains why, while isolated neutrons rapidly decay, a neutron inside an atomic nucleus is effectively stable. It cannot decay because no lower energy positions are available for the resultant proton. What if electrons were bosons rather than fermions? The result would be disastrous as there would be nothing to prevent all the electrons occupying the lowest level in an atom, like stuffing as many photons in the box as you can. Once again, wave chemistry – and the chemical complexity and flexibility needed by life – goodbye. These bosonic electrons would be very tightly bound to their nuclei, with little inclination to be shared with other atoms. This universe would be a sea of individual atoms floating through the cosmos, minding their own business and not getting involved in this messy work of forming molecules. However, the situation could be even more complicated! As well as the electrons, the quarks are also fermions, also possessing a spin of one-half. And this spin is imprinted (in a rather complex fashion) onto the particle they form. And both the proton and the neutron, even though they are composite objects, have a total spin of one-half, also making them fermions, and ensuring they obey the fermion rules of packing. This means that the protons and neutrons of an atom are arranged in orbits very similar to the orbits of the much more distant electrons. If quarks, and so also the protons and neutrons, had an integer spin, just like the electrons, there would be nothing to prevent all of them collapsing down and occupying the lowest energy level. Collapsing all of the protons and neutrons in your atoms is not necessarily a catastrophe; there are more important things to worry about.
If Pauli exclusion principle did not exist, we would not have a stable atom and hence no chemistry. Ethan Siegel calls this the biggest deal of all.
CALUM MILLER Defence of the fine-tuning argument JULY 25, 2017
Bohr’s Quantization Rule
Electrons can be seen as planet-like entities that orbit the nucleus but only at explicit energy levels that are governed by straightforward mathematical rules.
Danish physicist Niels Bohr proposed this at the beginning of the 20th century, suggesting that electrons can only occupy discrete orbitals around atoms. If this were not the case, then electrons would gradually reduce their energy (by radiation) and eventually (though very rapidly) lose their orbits. This would preclude atomic stability and chemical complexity, and so also preclude the existence of EMAs.
The Pauli Exclusion Principle
Newscientist (2014): Without the Pauli exclusion principle, matter as we know it would not exist. Named after the Austrian physicist Wolfgang Pauli, who proposed it in 1925, it says that no two electrons in an atom can enter the same quantum state. This leads to atomic electrons filling an elaborate structure of higher and higher energies around the nucleus, explaining why atoms with different numbers of electrons have different properties. Without the Pauli exclusion principle, chemical elements wouldn’t exist. The principle applies not just to electrons, but to all the particles that make up conventional matter, collectively known as fermions. Its influence extends even to the stars.
E.Siegel If it weren't for the Pauli Exclusion Principle, the matter we have in our Universe would behave in an extraordinarily different fashion. The electrons, you see, are examples of fermions. Every electron is fundamentally identical to every other electron in the Universe, with the same charge, mass, lepton number, lepton family number, and intrinsic angular momentum (or spin).
Princeton physicist Freeman Dyson has pointed out (1979, p. 251), if the Pauli exclusion principle did not exist—which is what keeps two electrons from occupying the same energy state in an atom—all electrons would occupy the lowest atomic energy state, and thus no complex atoms could exist. Thus, if any of these fundamental laws or principles were missing, the existence of complex, intelligent life would probably be rendered impossible.
This principle, formalized in 1925 by Austrian physicist Wolfgang Pauli, says that no two particles with half-integer spin (fermions) can occupy the same quantum state at the same time. Since each orbital has only two possible quantum states, this implies that only two electrons can occupy each orbital. This prevents electrons from all occupying the lowest atomic orbital, and so facilitates complex chemistry
https://calumsblog.com/2017/07/25/full-defence-of-the-fine-tuning-argument-part-4/
Finally, consider the Pauli Exclusion Principle, which dictates that no two fermions (spin-½ particles) can occupy the same quantum state. This arises from a deep principle in quantum mechanics which requires that the joint wave function of a system of fermions be antisymmetric. This implies that not more than two electrons can occupy the same orbital in an atom, since a single orbital consists of two possible quantum states (or more precisely, eigenstates) corresponding to the spin pointing in one direction and the spin pointing in the opposite direction. This allows for complex chemistry since without this principle, all electrons would occupy the lowest atomic orbital. Thus, without this principle, no complex life would be possible.
https://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.696.63&rep=rep1&type=pdf
Luke A. Barnes The Fine-Tuning of the Universe for Intelligent Life June 11, 2012
If electrons were bosons, rather than fermions, then they would not obey the Pauli exclusion principle. There would be no chemistry.
https://arxiv.org/pdf/1112.4647.pdf
Stephen C. Meyer: The return of the God hypothesis, page 189
The operation of a principle in the physical world such as the Pauli exclusion principle that (a) enables complex material structures to form and yet (b) limits the atomic weight of elements (by limiting the number of neutrons in the lowest nuclear shell). Thus, the forces at work in the universe itself (and the mathematical laws of physics describing them) display a fine-tuning that requires explanation. Yet, clearly, no physical explanation of this structure is possible, because it is precisely physics (and its most fundamental laws) that manifests this structure and requires explanation. Indeed, clearly physics does not explain itself. See Gordon, “Divine Action and the World of Science,” esp. 258–59; Collins, “The Fine-Tuning Evidence Is Convincing,” esp. 36–38.
If electrons were bosons, rather than fermions, then they would not obey the Pauli exclusion principle. There would be no chemistry. It implies that not more than two electrons can occupy the same orbital in an atom, since a single orbital consists of two possible quantum states corresponding to the spin pointing in one direction and the spin pointing in the opposite direction. This allows for complex chemistry since, without this principle, all electrons would occupy the lowest atomic orbital. Thus, without this principle, no complex life would be possible.
R. P. Feynman wrote of the Pauli exclusion principle, “In fact, almost all the peculiarities of the material world hinge on this wonderful fact.” Measurements show no violation and confirm the generalized Pauli exclusion principle with an error of one part in one quintillion.
https://www.nature.com/articles/s42005-019-0110-3
S. Hossenfelder (2020): The Pauli exclusion principle is a law of nature; it's just how the world is.
https://www.frontiersin.org/articles/10.3389/fphy.2020.00139/full
Electrons in an atom form what are known as standing waves, which just means a wave confined in a certain area. Electrons in atoms form three-dimensional standing waves called spherical harmonics.

